mathematical fact that the area of a two-dimensional circle is π r2, while the volume of a three-dimensional sphere is 4/3 π r3, the volume of a four-dimensional hypersphere is 1/2 π2 r4, and so on.
Below I show pictures of various networks. In each case the first picture is drawn to emphasize obvious regularities in the network. But the second picture is drawn in a more systematic way—by picking a specific starting node, and then laying out other nodes so that those at
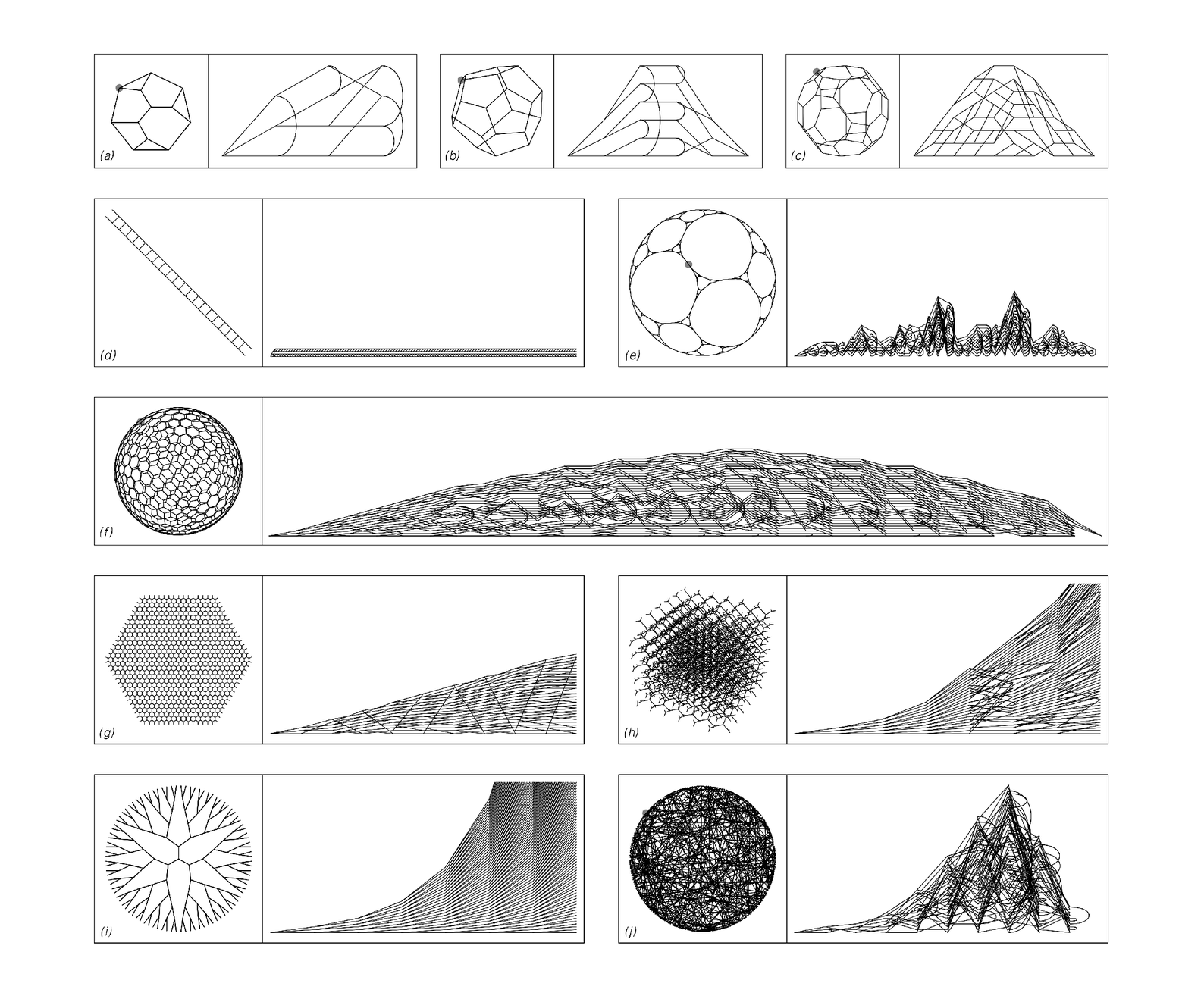
Examples of various networks, shown first to emphasize their regularities, and second to illustrate the number of nodes reached by going successively more steps from a given node. For networks that in a limiting sense correspond to ordinary d-dimensional space, this number grows like rd-1. All the larger networks shown are approximately uniform, in the sense that similar results are obtained starting from any node. Network (e) effectively has limiting dimension Log[2,3]≃1.58.



