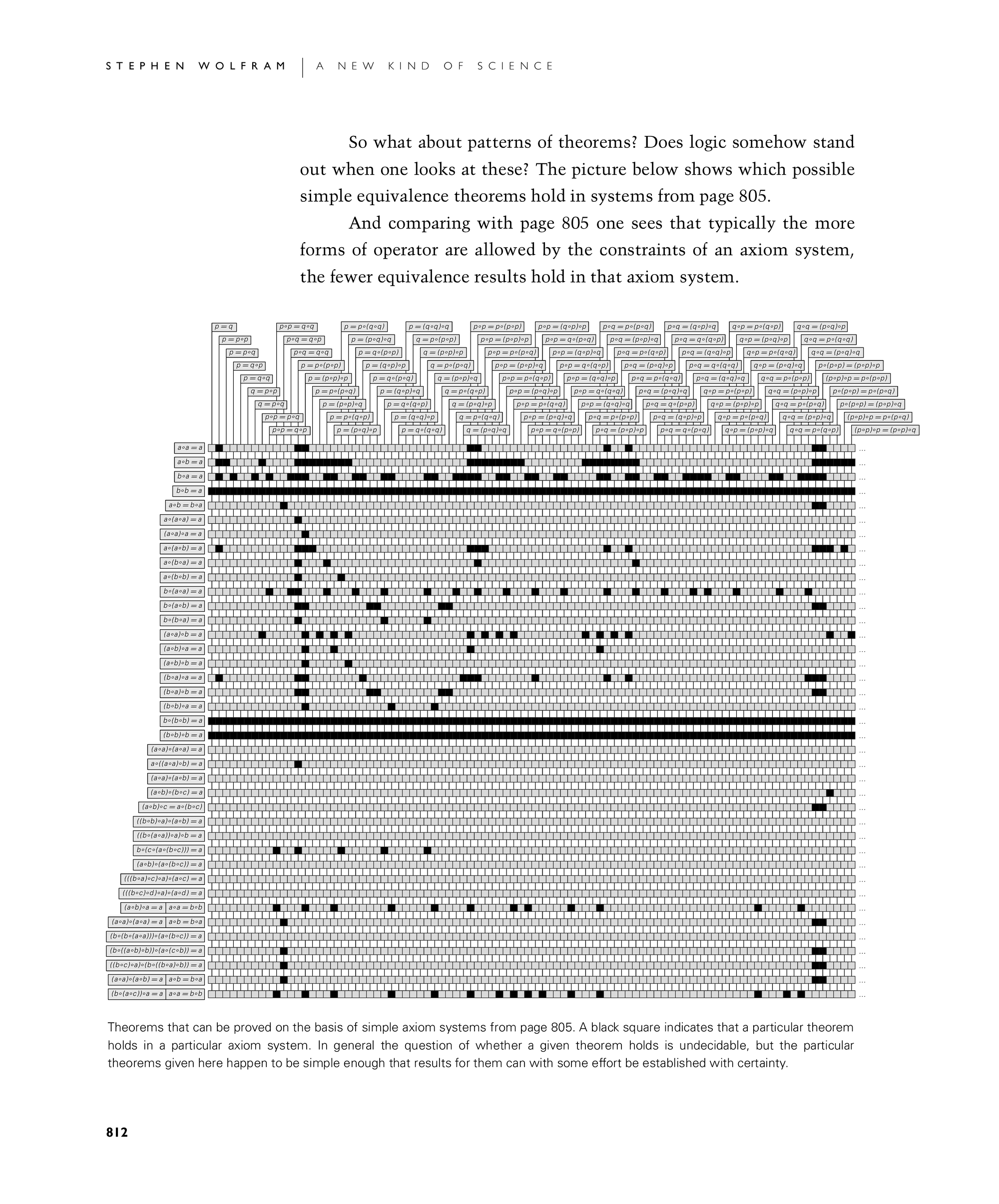
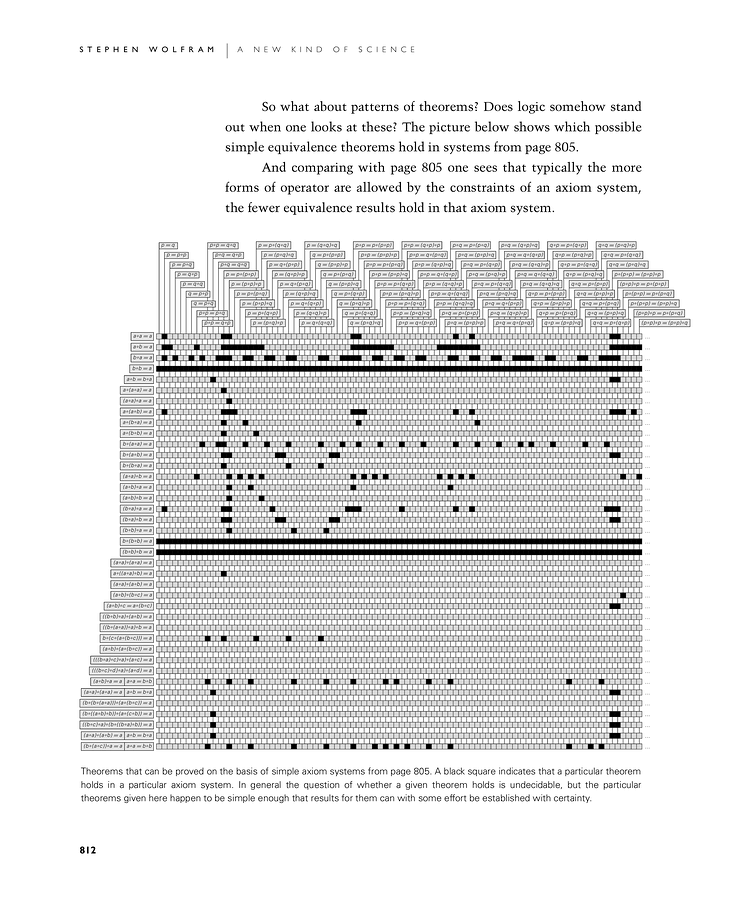
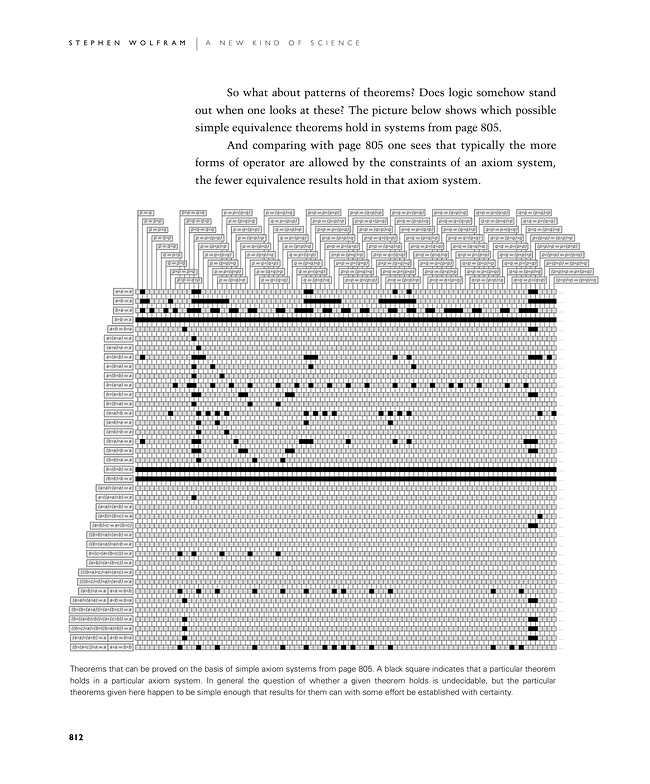
So what about patterns of theorems? Does logic somehow stand out when one looks at these? The picture below shows which possible simple equivalence theorems hold in systems from page 805.

And comparing with page 805 one sees that typically the more forms of operator are allowed by the constraints of an axiom system, the fewer equivalence results hold in that axiom system.

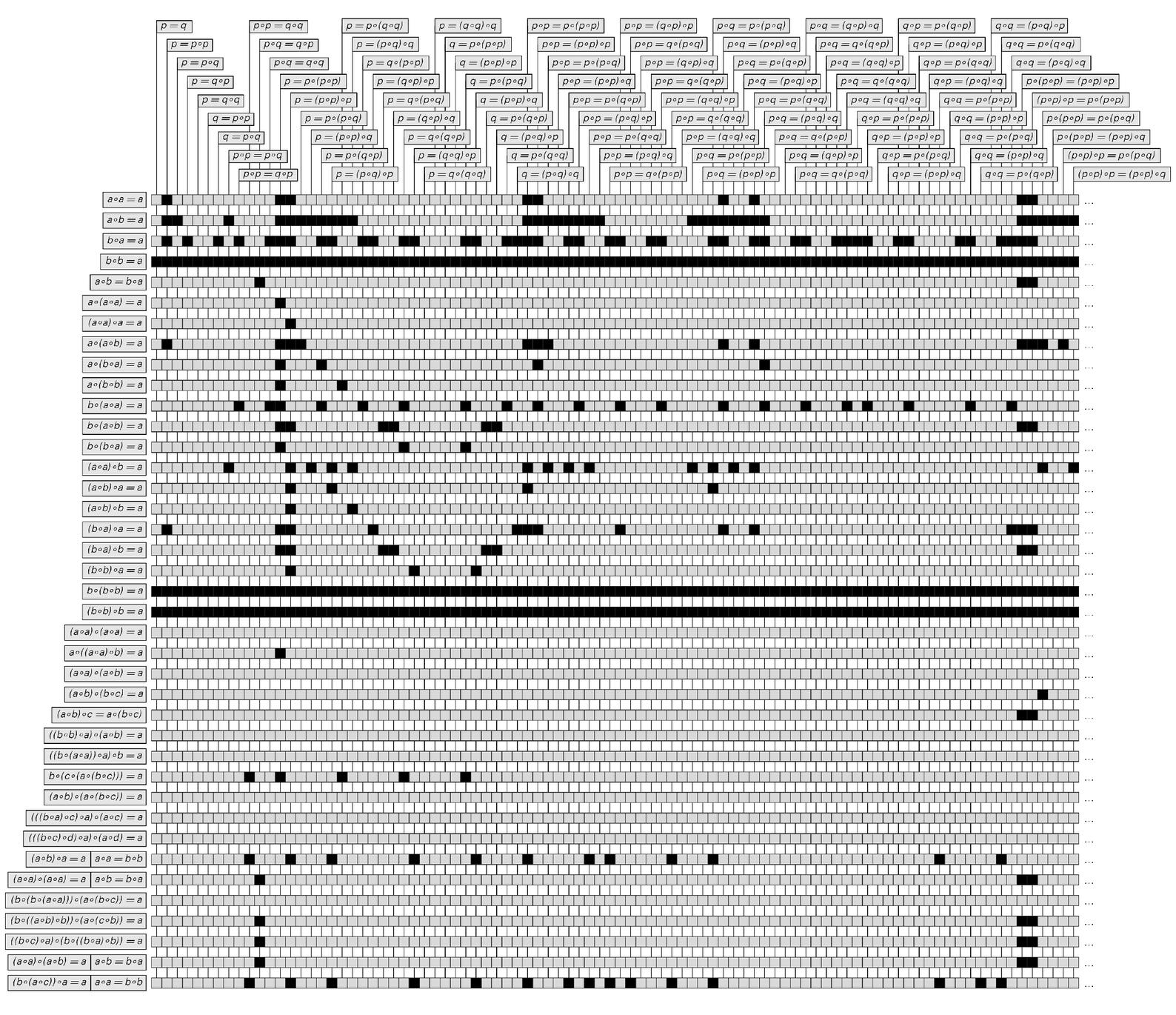
Theorems that can be proved on the basis of simple axiom systems from page 805. A black square indicates that a particular theorem holds in a particular axiom system. In general the question of whether a given theorem holds is undecidable, but the particular theorems given here happen to be simple enough that results for them can with some effort be established with certainty.