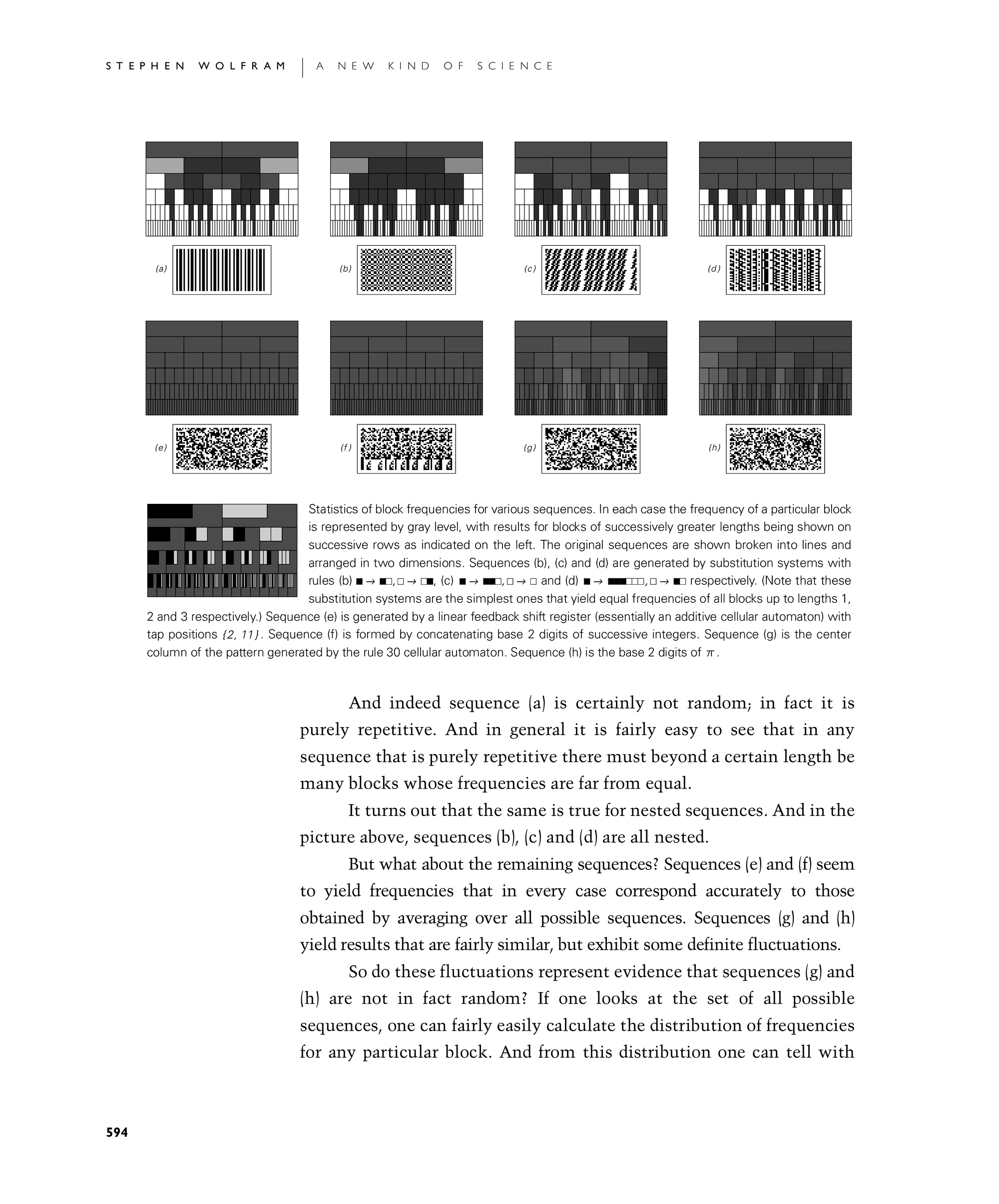
And indeed sequence (a) is certainly not random; in fact it is purely repetitive. And in general it is fairly easy to see that in any sequence that is purely repetitive there must beyond a certain length be many blocks whose frequencies are far from equal.

It turns out that the same is true for nested sequences. And in the picture below, sequences (b), (c) and (d) are all nested.

But what about the remaining sequences? Sequences (e) and (f) seem to yield frequencies that in every case correspond accurately to those obtained by averaging over all possible sequences. Sequences (g) and (h) yield results that are fairly similar, but exhibit some definite fluctuations.

So do these fluctuations represent evidence that sequences (g) and (h) are not in fact random? If one looks at the set of all possible sequences, one can fairly easily calculate the distribution of frequencies for any particular block. And from this distribution one can tell with

Statistics of block frequencies for various sequences. In each case the frequency of a particular block is represented by gray level, with results for blocks of successively greater lengths being shown on successive rows as indicated on the bottom left. The original sequences are shown broken into lines and arranged in two dimensions. Sequences (b), (c) and (d) are generated by substitution systems with rules (b) ,, (c) , and (d) , respectively. (Note that these substitution systems are the simplest ones that yield equal frequencies of all blocks up to lengths 1, 2 and 3 respectively.) Sequence (e) is generated by a linear feedback shift register (essentially an additive cellular automaton) with tap positions {2, 11}. Sequence (f) is formed by concatenating base 2 digits of successive integers. Sequence (g) is the center column of the pattern generated by the rule 30 cellular automaton. Sequence (h) is the base 2 digits of π.