that a digit which appears at a particular position in their result can depend on digits that were originally far away from it.

But despite fundamental differences like this in underlying rules, the overall behavior produced by systems based on numbers is still very similar to what one sees for example in cellular automata.

So just like for the various kinds of programs that we discussed in the previous chapter, the details of underlying rules again do not seem to have a crucial effect on the kinds of behavior that can occur.

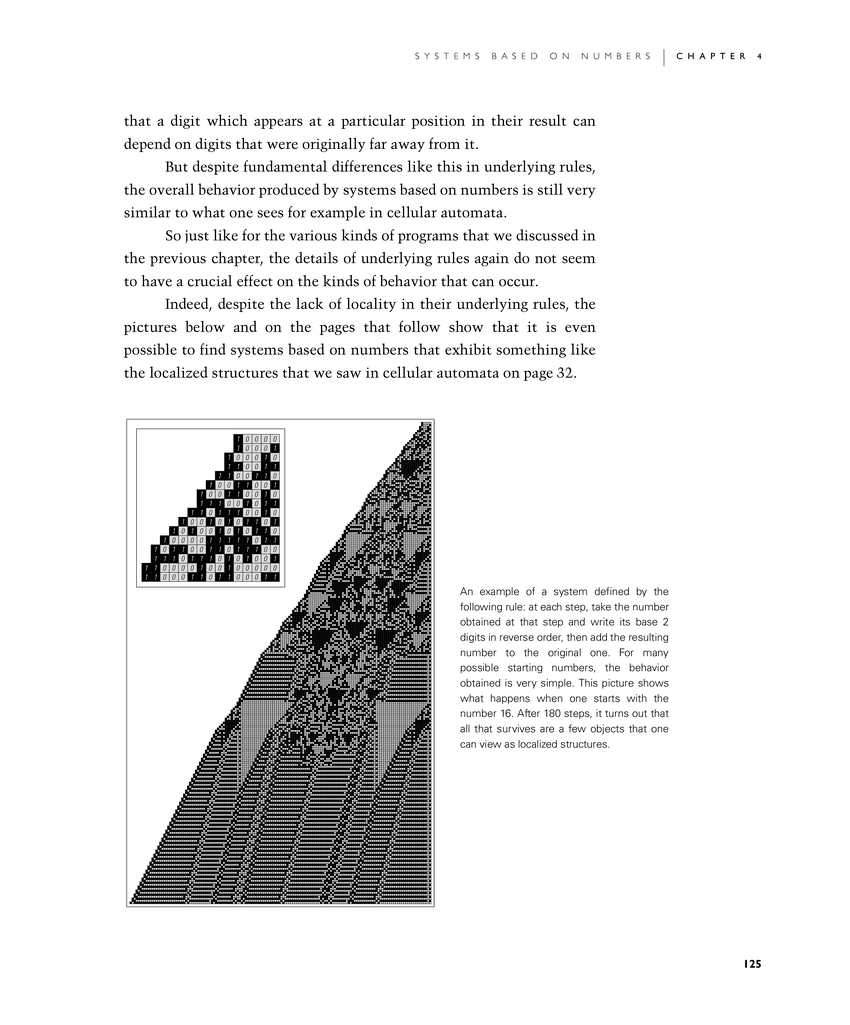
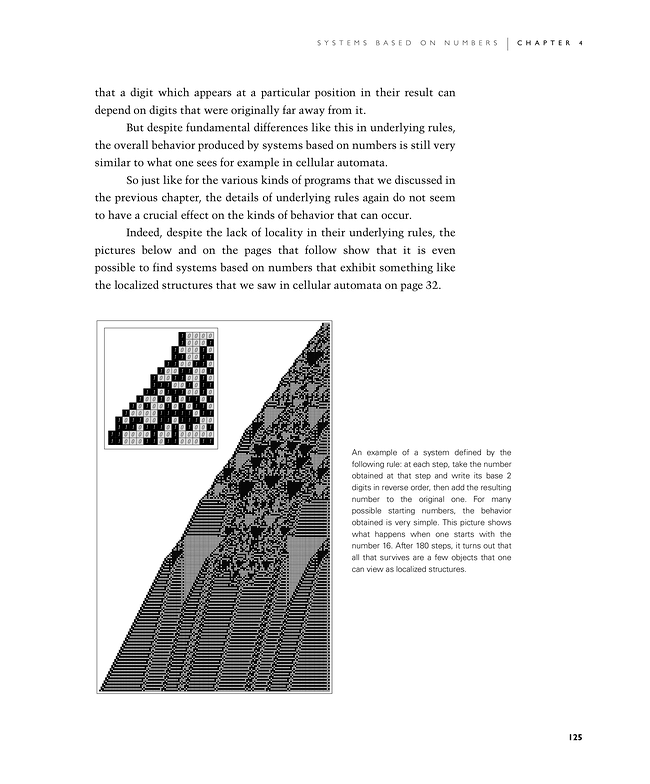
Indeed, despite the lack of locality in their underlying rules, the pictures below and on the pages that follow [126, 127] show that it is even possible to find systems based on numbers that exhibit something like the localized structures that we saw in cellular automata on page 32.

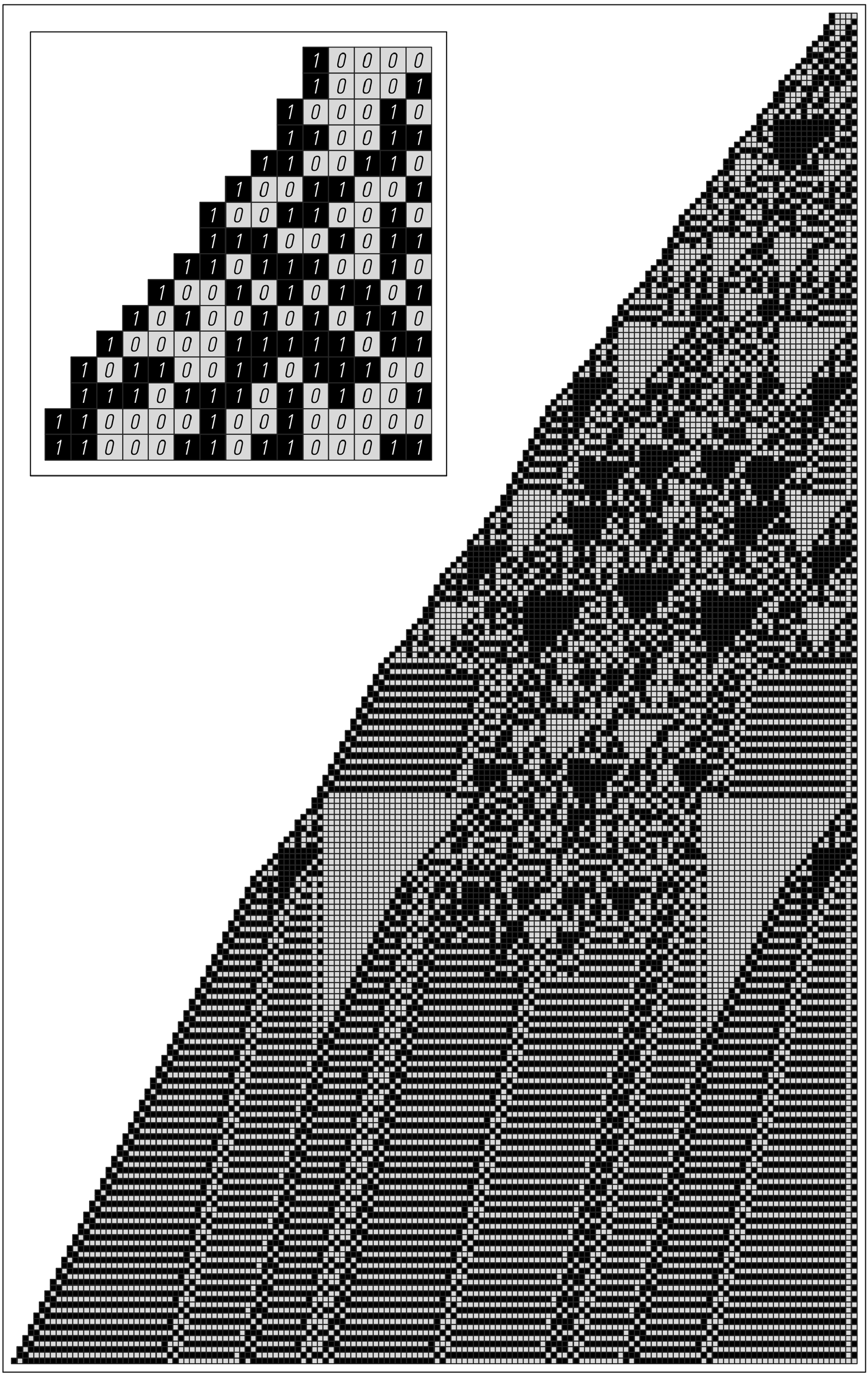
An example of a system defined by the following rule: at each step, take the number obtained at that step and write its base 2 digits in reverse order, then add the resulting number to the original one. For many possible starting numbers, the behavior obtained is very simple. This picture shows what happens when one starts with the number 16. After 180 steps, it turns out that all that survives are a few objects that one can view as localized structures.