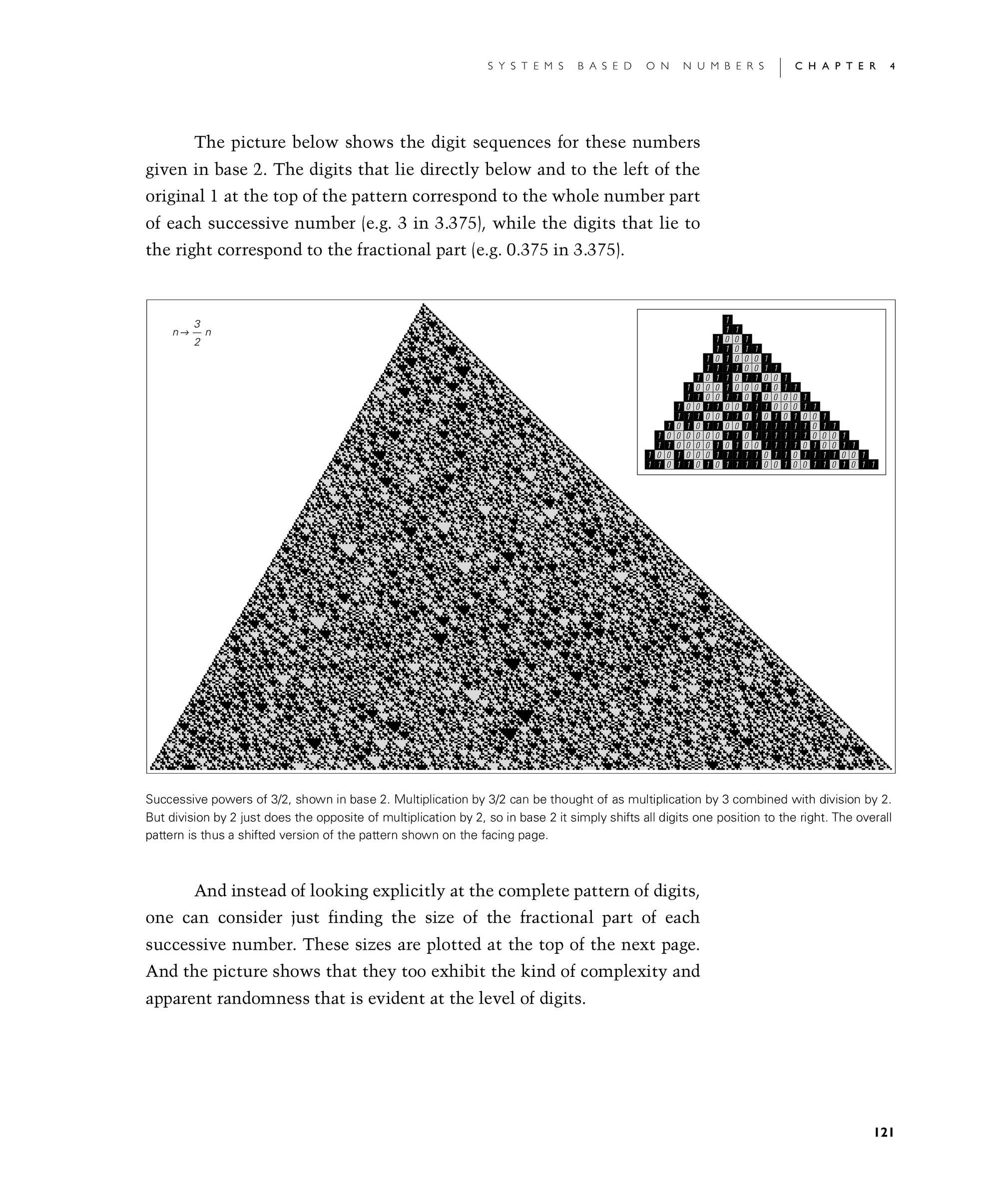
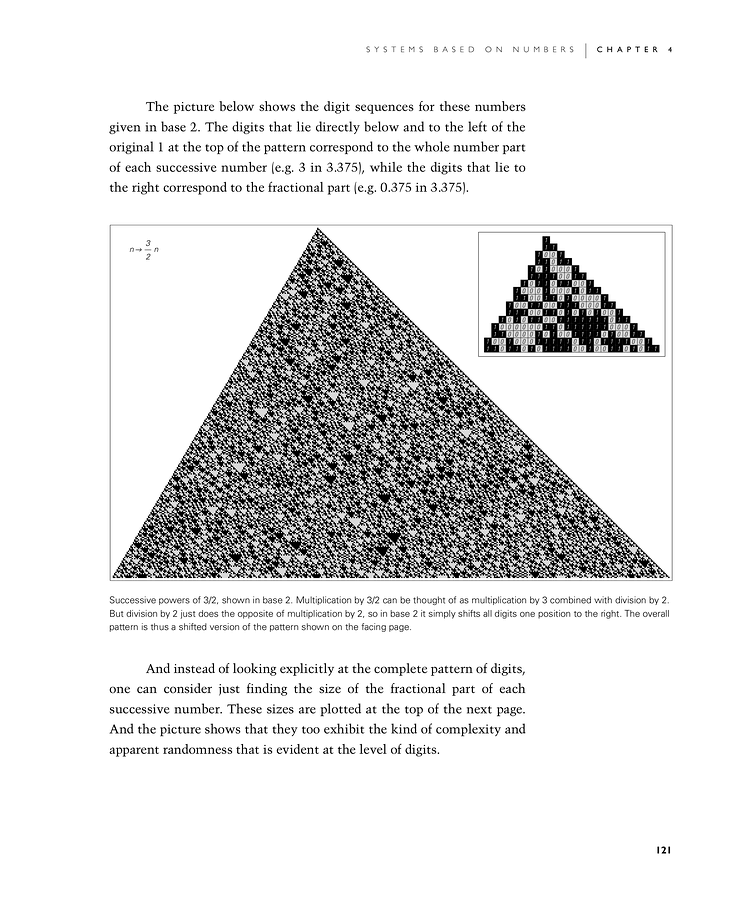
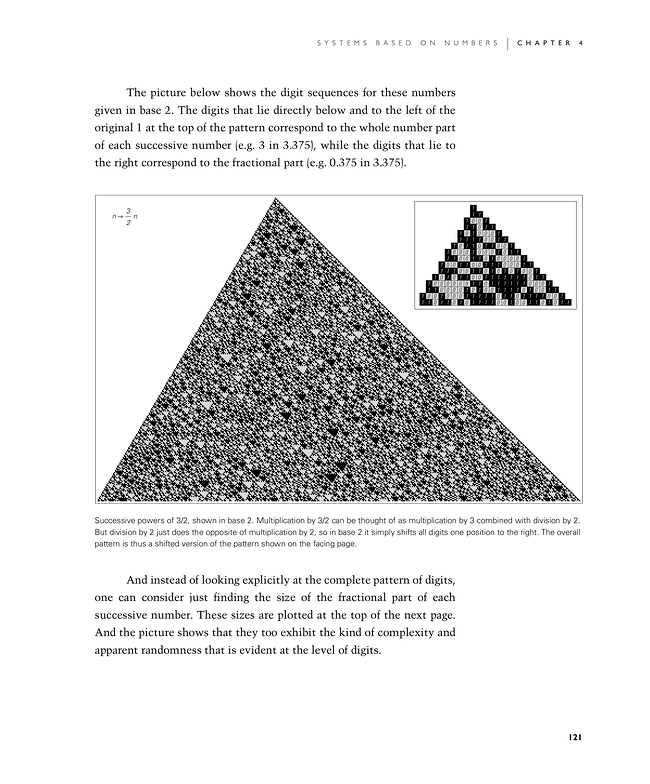
The picture below shows the digit sequences for these numbers given in base 2. The digits that lie directly below and to the left of the original 1 at the top of the pattern correspond to the whole number part of each successive number (e.g. 3 in 3.375), while the digits that lie to the right correspond to the fractional part (e.g. 0.375 in 3.375).

And instead of looking explicitly at the complete pattern of digits, one can consider just finding the size of the fractional part of each successive number. These sizes are plotted at the top of the next page. And the picture shows that they too exhibit the kind of complexity and apparent randomness that is evident at the level of digits.

Successive powers of 3/2, shown in base 2. Multiplication by 3/2 can be thought of as multiplication by 3 combined with division by 2. But division by 2 just does the opposite of multiplication by 2, so in base 2 it simply shifts all digits one position to the right. The overall pattern is thus a shifted version of the pattern shown on the facing page.