There are typically many different ways to draw a non-planar network, each with lines crossing in different places. But there is a fundamental result in graph theory that shows that if a network is not planar, then it must always be possible to identify in it a specific part that can be reduced to one of the two forms shown in the top picture—or just the second form for a network with three connections at each node.
So this implies that one can in fact meaningfully associate a definite structure with non-planarity. And while at some level the structure can be spread out in the network, the point is that it must always in effect have a localized core with the form shown in the second picture.
In general one can imagine having several pieces of non-planarity in a network—perhaps each pictured like a carrying handle. But if the underlying rules for the network preserve planarity then each of these pieces of non-planarity must on their own be persistent—and can in a sense only disappear through processes like annihilating with each other.
So might these be like actual particles in physics?
In the realistic case of network rules for the universe, planarity as such is presumably not preserved. But observations in physics suggest that there are several quantities like electric charge that are conserved. And ultimately the values of these quantities must reflect properties of underlying networks that are preserved by network evolution rules.
And if these rules satisfy the constraint of causal invariance that I discussed in previous sections [10, 11, 12, 13], then I suspect that this means that they will inevitably exhibit various additional features—perhaps notably including for example what is usually known as local gauge invariance.
But what is most relevant here is that it seems likely that—much as for non-planarity—nonzero values of quantities conserved by network evolution rules can be thought of as being associated with some sort of local structures or tangles of connections in the network. And I suspect that it is essentially such structures that define the cores of the various types of elementary particles that are seen in physics.
Before the results of this book it might have seemed completely implausible that anything like this could be correct. For independent of any specific arguments about networks and their evolution, traditional intuition would tend to make one think that the elaborate properties of
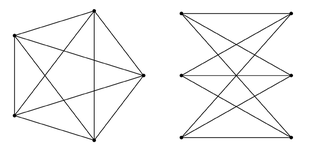
The K5 and K3,3 forms that lead to non-planarity in networks.

How K3,3 is embedded in the network from the facing page.