Financial Systems

During the development of the ideas in this book I have been asked many times whether they might apply to financial systems. There is no doubt that they do, and as one example I will briefly discuss here what is probably the most obvious feature of essentially all financial markets: the apparent randomness with which prices tend to fluctuate.

Whether one looks at stocks, bonds, commodities, currencies, derivatives or essentially any other kind of financial instrument, the sequences of prices that one sees at successive times show some overall trends, but also exhibit varying amounts of apparent randomness.

So what is the origin of this randomness?

In the most naive economic theory, price is a reflection of value, and the value of an asset is equal to the total of all future earnings—such as dividends—which will be obtained from it, discounted for the interest that will be lost from having to wait to get these earnings.

With this view, however, it seems hard to understand why there should be any significant fluctuations in prices at all. What is usually said is that prices are in fact determined not by true value, but rather by the best estimates of that value that can be obtained at any given time. And it is then assumed that these estimates are ultimately affected by all sorts of events that go on in the world, making random movements

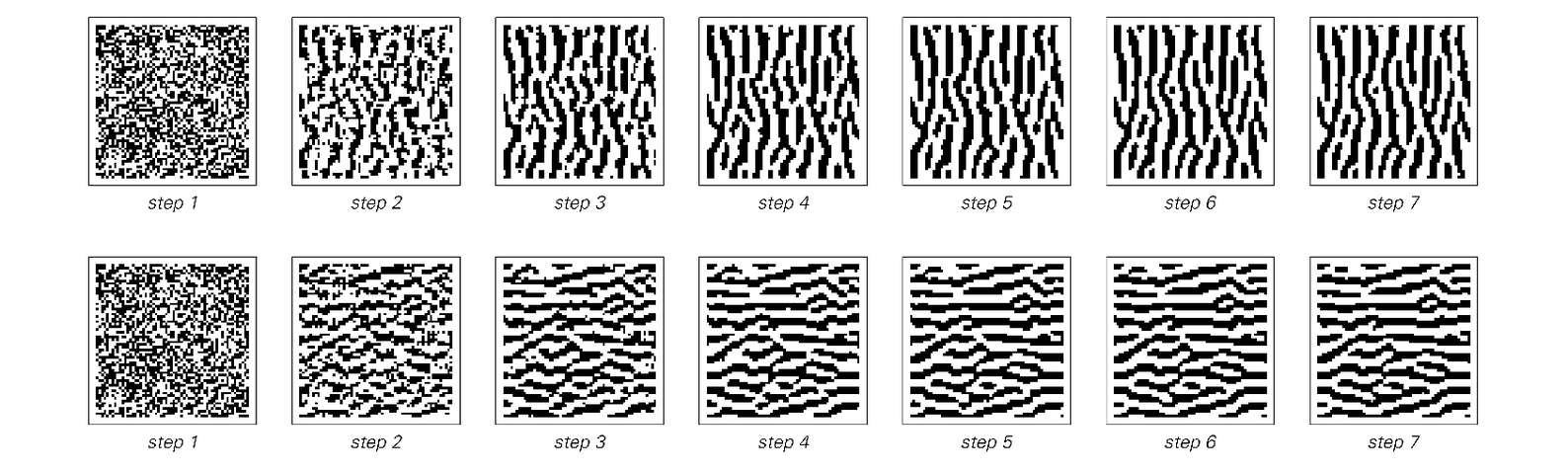
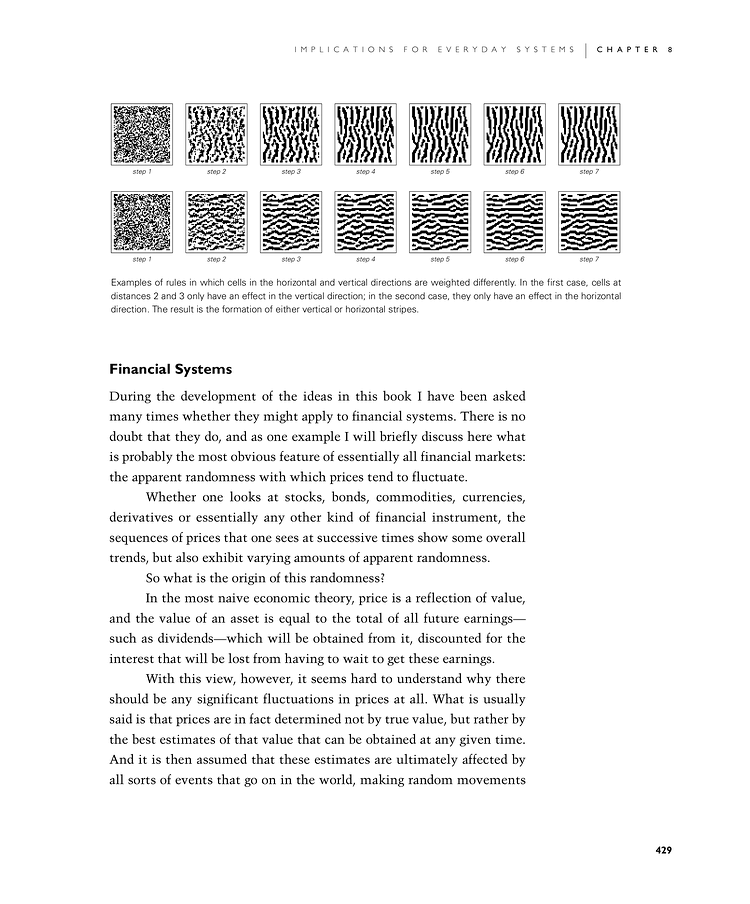
Patterns generated by rules of the type shown on the previous page, with a range of choices for the weights of cells at distances 2 and 3. Weights vary from -0.9 to 0 down the page for distance 2, and from -0.7 to 0.4 across the page for distance 3. In all cases the evolution starts from the same random initial condition, and is continued until it stabilizes. Note that pigmentation patterns for actual animals may contain either larger or smaller numbers of elements than the patterns shown here.