this means is that with the setup used the underlying axiom system is inconsistent. So what about the other multiway systems on the facing page? At least with the strings one can see in the pictures there are no inconsistencies. But what about with longer strings? For the particular rules shown it is fairly easy to demonstrate that there are never inconsistencies. But in general it is not possible to do this, for after some given string has appeared, it can for example be undecidable whether the negation of that particular string ever appears.

So what about the axiom systems normally used in actual mathematics? None of those on pages 773 and 774 appear to be inconsistent. And what this means is that the set of statements that can be proved true will never overlap with the set that can be proved false.

But can every possible statement that one might expect to be true or false actually in the end be proved either true or false?

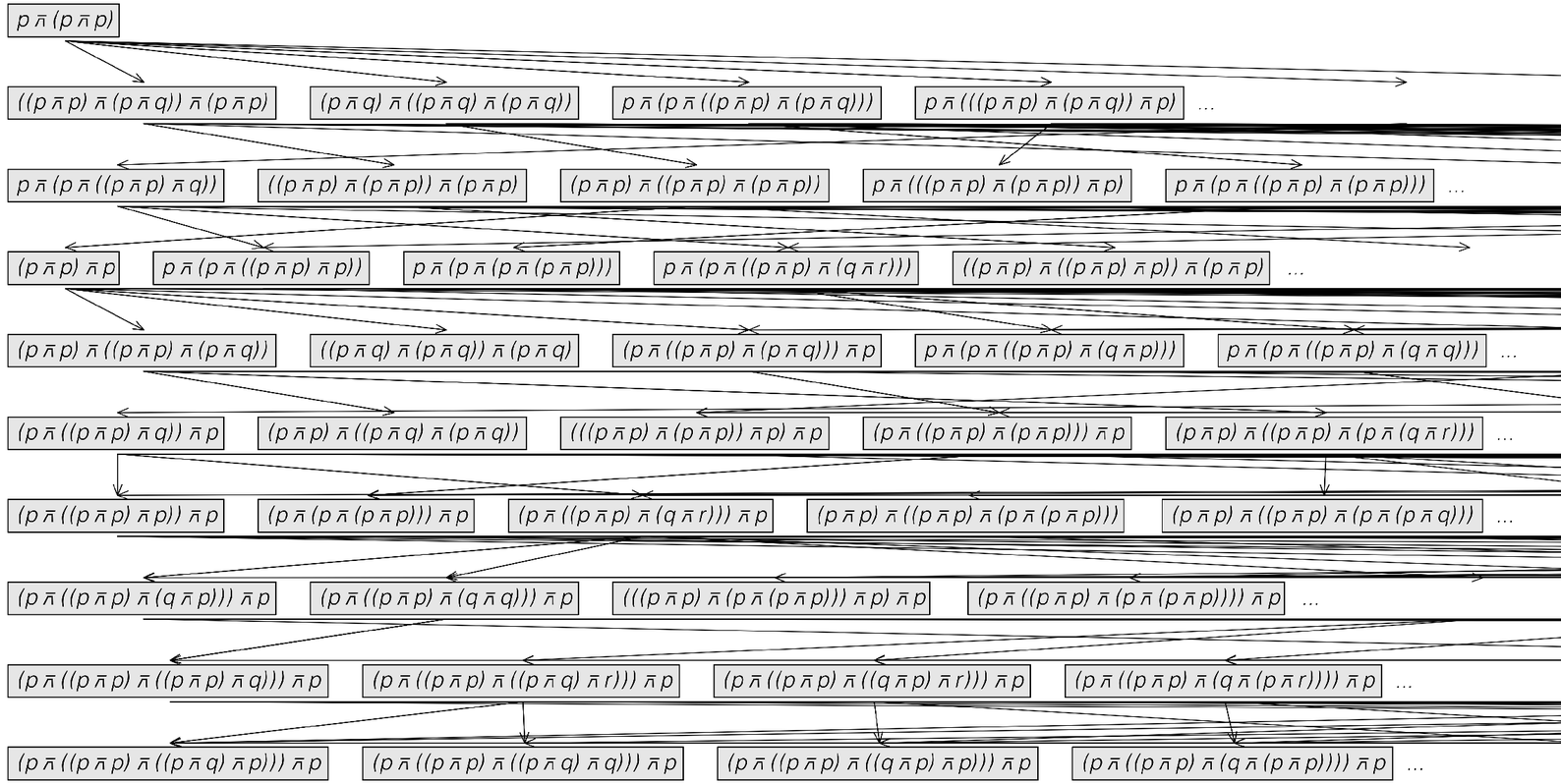
The network of statements that can be proved true using the axiom system for logic from page 775. p ⊼ (p ⊼ p) is the simplest representation for True when logic is set up using the Nand operator ⊼. Each arrow indicates an equivalence established by applying a single axiom. On each row only statements that have not appeared before are given. The statements are sorted so that the simplest are first. Note that some fairly simple statements do not show up for at least several rows. The total number of statements on successive rows grows faster than exponentially; for the first few it is 1, 6, 91, 2180, 76138. If continued forever the network would eventually include all possible true statements (tautologies) of logic (see also page 818). Other simple axiom systems for logic like those on page 808 yield networks similar to the one shown.