always seem to move at the same speed, and so can never interact. And even after searching many billions of cases, I have never succeeded in finding any useful set of localized structures in rule 30.

The picture below shows what happens in rule 45. Many possible perturbations to repetitive initial conditions again yield seemingly random behavior. But in one case a nested pattern is produced. And structures that remain localized are now fairly common—but just as in rule 30 always seem to move at the same speed.

So although this means that the particular type of approach we used to demonstrate the universality of rule 110 cannot immediately be used for rule 30 or rule 45, it certainly does not mean that these rules are not in the end universal. And as I will discuss in the next chapter, it is my very strong belief that in fact they will turn out to be.

So how might we get evidence for this?

If a system is universal, then this means that with a suitable encoding of initial conditions its evolution must emulate the evolution of any other system. So this suggests that one might be able to get evidence about universality just by trying different possible encodings, and then seeing what range of other systems they allow one to emulate.

In the case of the 19-color universal cellular automaton on page 645 it turns out that encodings in which individual black and white cells are represented by particular 20-cell blocks are sufficient to allow the universal cellular automaton to emulate all 256 possible elementary cellular automata—with one step in the evolution of each of these corresponding to 53 steps in the evolution of the original system.

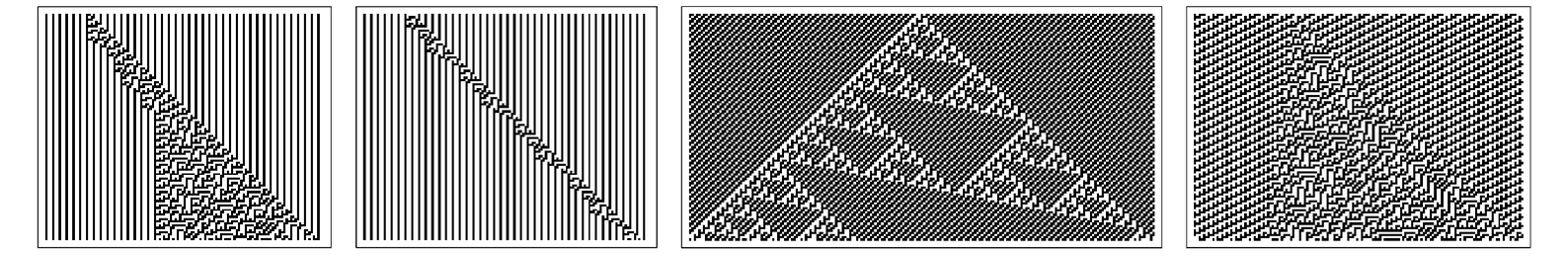
Examples of patterns produced by inserting a single extra black cell into repetitive backgrounds for rule 45. Note the appearance of a slanted version of the nested pattern from rule 90. In rule 45, localized structures turn out to be fairly common—but as in rule 30 they always seem to move at the same speed, and so presumably cannot interact to produce any kind of class 4 behavior.