The Threshold of Universality in Cellular Automata

By showing that rule 110 is universal, we have established that universality is possible even among cellular automata with the very simplest kinds of underlying rules. But there remains the question of what is ultimately needed for a cellular automaton—or any other kind of system—to be able to achieve universality.

In general, if a system is to be universal, then this means that by setting up an appropriate choice of initial conditions it is possible to get the system to emulate any type of behavior that can occur in any other system. And as a consequence, cellular automata like the ones in the pictures below are definitely not universal, since they always produce just simple uniform or repetitive patterns of behavior, whatever initial conditions one uses.

In a sense the fundamental reason for this—as we discussed on page 252—is that such class 1 and class 2 cellular automata never allow any transmission of information except over limited distances. And the result of this is that they can only support processes that involve the correlated action of a limited number of cells.

In cellular automata like the ones at the top of the facing page some information can be transmitted over larger distances. But the way this occurs is highly constrained, and in the end these systems can only produce patterns that are in essence purely nested—so that it is again not possible for universality to be achieved.

What about additive rules such as 90 and 150?

With simple initial conditions these rules always yield very regular nested patterns. But with more complicated initial conditions, they produce more complicated patterns of behavior—as the second set of pictures at

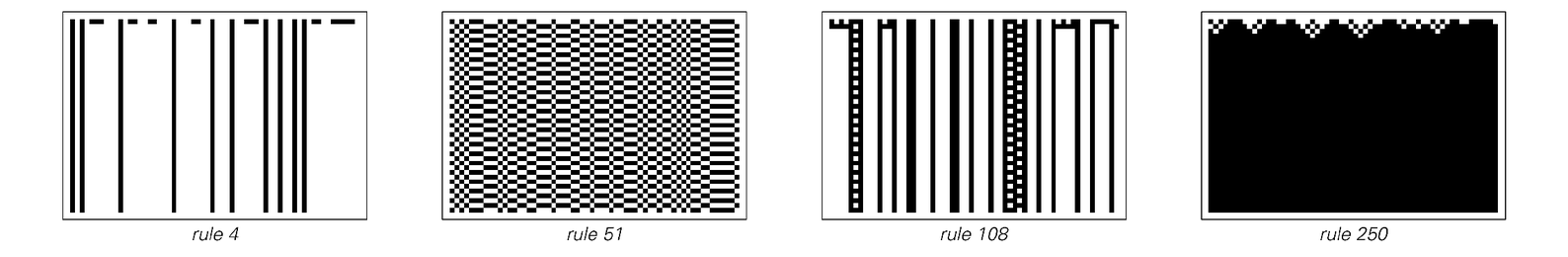
Examples of elementary cellular automata which only ever show purely uniform or purely repetitive behavior, and which therefore definitely cannot be universal. These cellular automata are necessarily all class 1 or class 2 systems.