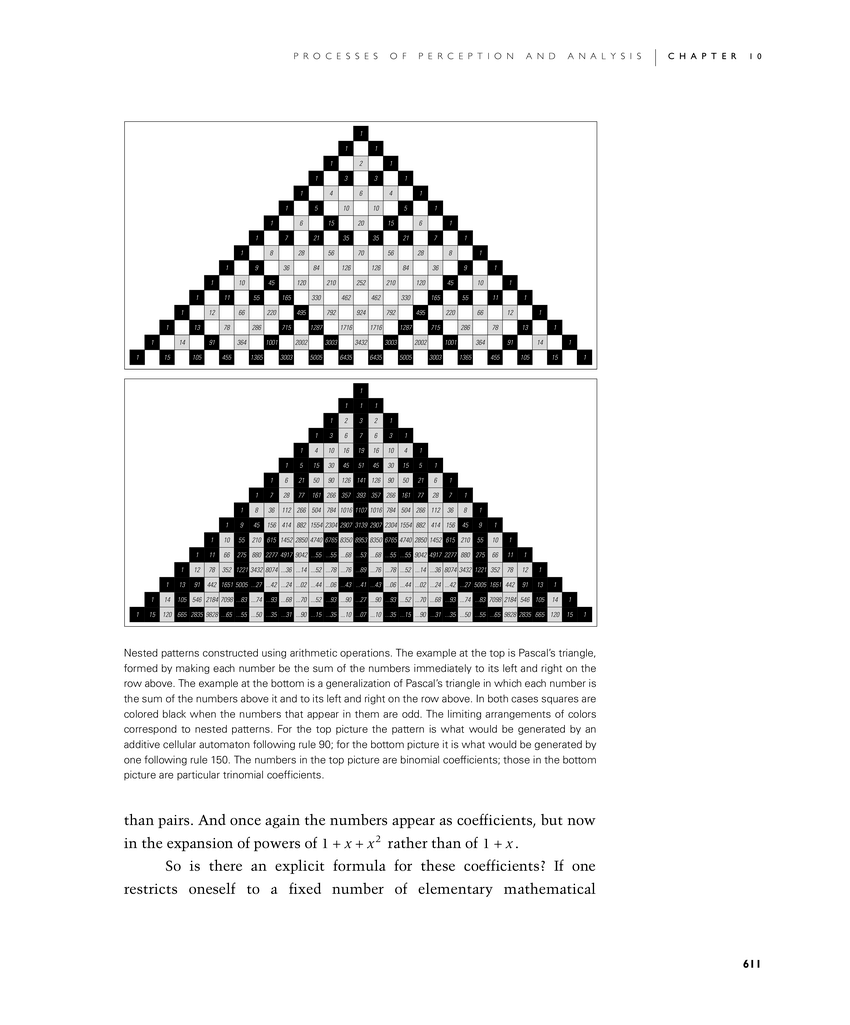
than pairs. And once again the numbers appear as coefficients, but now in the expansion of powers of 1 + x + x² rather than of 1 + x.

So is there an explicit formula for these coefficients? If one restricts oneself to a fixed number of elementary mathematical

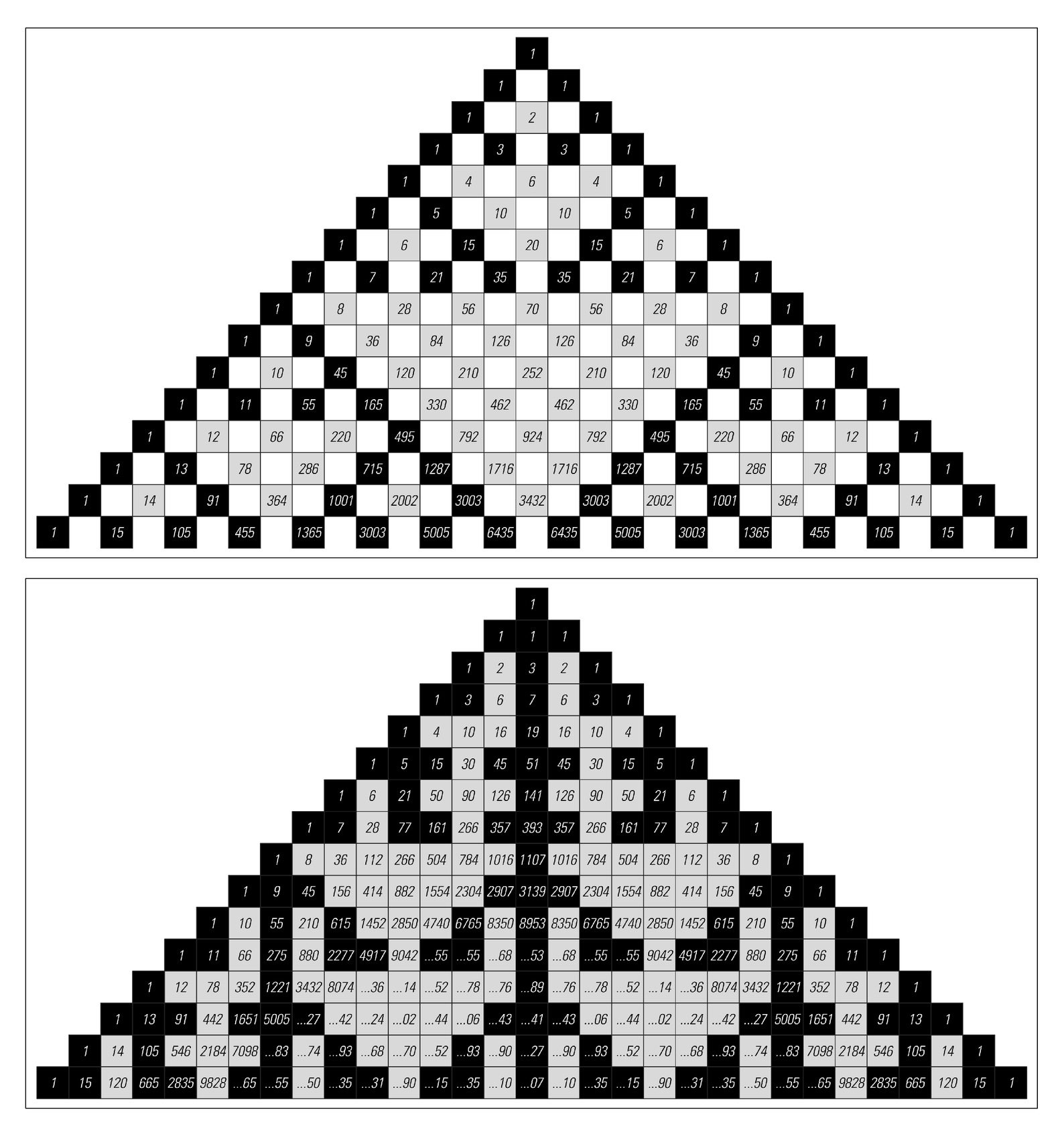
Nested patterns constructed using arithmetic operations. The example at the top is Pascal's triangle, formed by making each number be the sum of the numbers immediately to its left and right on the row above. The example at the bottom is a generalization of Pascal's triangle in which each number is the sum of the numbers above it and to its left and right on the row above. In both cases squares are colored black when the numbers that appear in them are odd. The limiting arrangements of colors correspond to nested patterns. For the top picture the pattern is what would be generated by an additive cellular automaton following rule 90; for the bottom picture it is what would be generated by one following rule 150. The numbers in the top picture are binomial coefficients; those in the bottom picture are particular trinomial coefficients.