only at overall features, then typically one would tend to say that the third picture seems more complex than the other two.

For even though the detailed placement of black and white cells in the first two pictures does not seem simple to describe, at an overall level these pictures still admit a quite simple description: in essence they just involve a kind of uniform randomness in which every region looks more or less the same as every other. But the third picture shows no such uniformity, even at an overall level. And as a result, we cannot give a short description of it even if we ignore its small-scale details.

Of course, if one goes to an extreme and looks, say, only at how big each picture is, then all three pictures have very short descriptions. And in general how short a description of something one can find will depend on what features of it one wants to capture—which is why one may end up ascribing a different complexity to something when one looks at it for different purposes.

But if one uses a particular method of perception or analysis, then one can always see how short a description this manages to produce. And the shorter the description is, the lower one considers the complexity to be.

But to what extent is it possible to define a notion of complexity that is independent of the details of specific methods of perception and analysis? In this chapter I argue that essentially all common forms of perception and analysis correspond to rather simple programs. And if one is interested in descriptions in which no information is lost—as in the discussion of randomness in the previous section—then as I

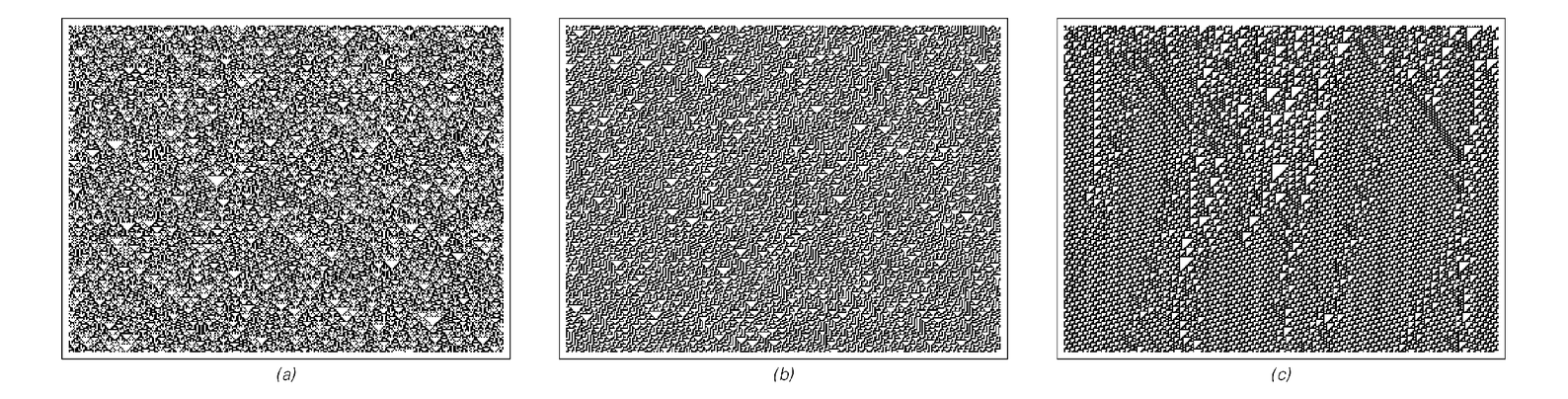
Examples of pictures that at an everyday level one might typically describe either as being "quite random" or as being "quite complex".