pictures, we can readily construct much shorter—yet still complete—descriptions of these pictures.

The repetitive structure of picture (a) implies that to reproduce this picture all we need do is to specify the colors in a 49×2 block, and then say that this block should be repeated an appropriate number of times. Similarly, the nested structure of picture (b) implies that to reproduce this picture, all we need do is to specify the colors in a 3×3 block, and then say that as in a two-dimensional substitution system each black cell should repeatedly be replaced by this block.

But what about picture (c)? Is there any short description that can be given of this picture? Or do we have no choice but just to specify explicitly the color of every one of the cells it contains?

Our powers of visual perception certainly do not reveal any significant regularities that would allow us to construct a shorter description. And neither, it turns out, do any standard methods of mathematical or statistical analysis. And so for practical purposes we have little choice but just to specify explicitly the color of each cell.

But the fact that no short description can be found by our usual processes of perception and analysis does not in any sense mean that no such description exists at all. And indeed, as it happens, picture (c) in fact allows a very short description. For it can be generated just by

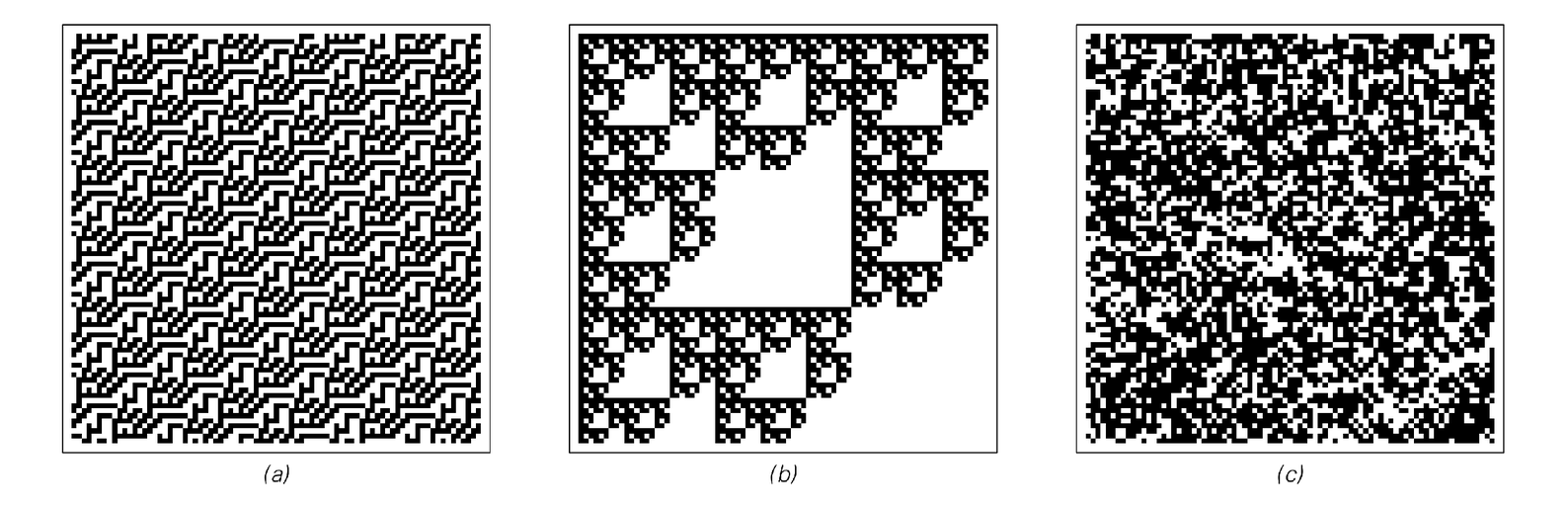
Pictures exhibiting different degrees of apparent randomness. Pictures (a) and (b) have obvious regularities, and would never be considered particularly random. But picture (c) has almost no obvious regularities, and would typically be considered quite random. As it turns out, picture (c), like (a) and (b), can actually be generated by a quite simple process. But the point is that the simplicity of this process does not affect the fact that with our standard methods of perception and analysis picture (c) is for practical purposes random.