As the embryo grows, however, the leg region will eventually become large enough that it can differentiate into several separate regions. And at this point, a distinct foot region can appear. Then, when the foot region becomes large enough, it too can break into separate regions that will, say, turn into bone or soft tissue. And when a region that will turn into bone becomes large enough, it can break into further regions that will, say, yield separate individual bones.

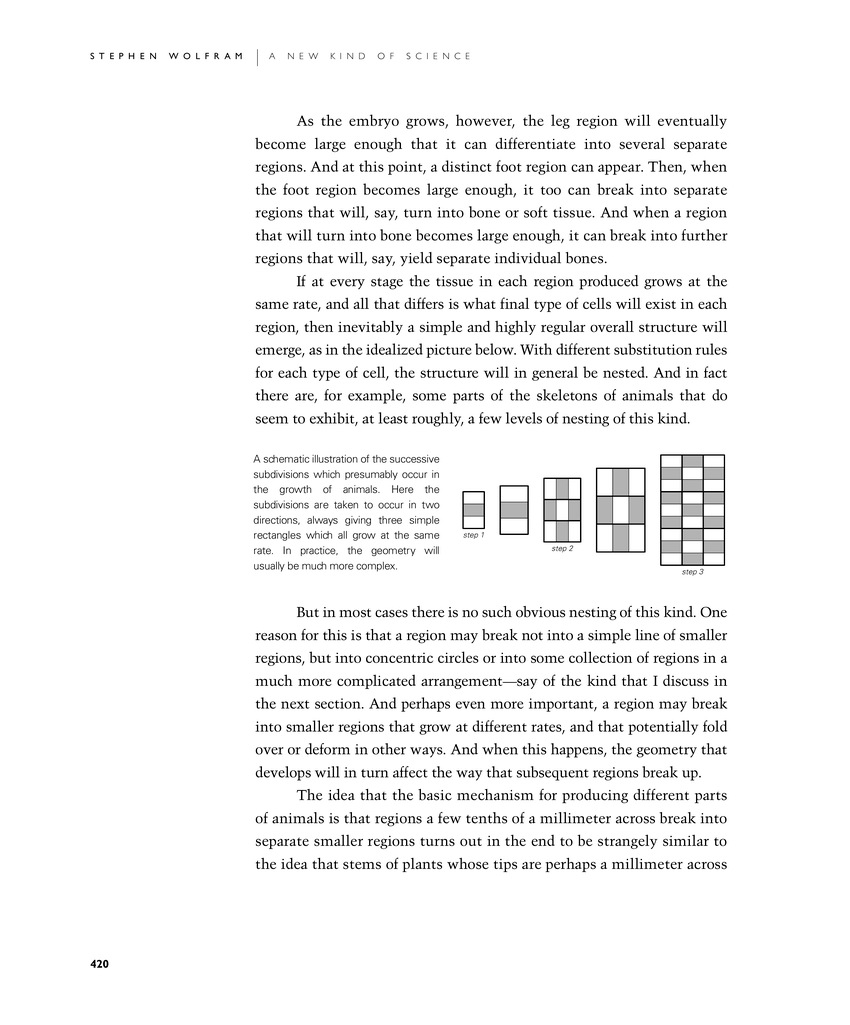
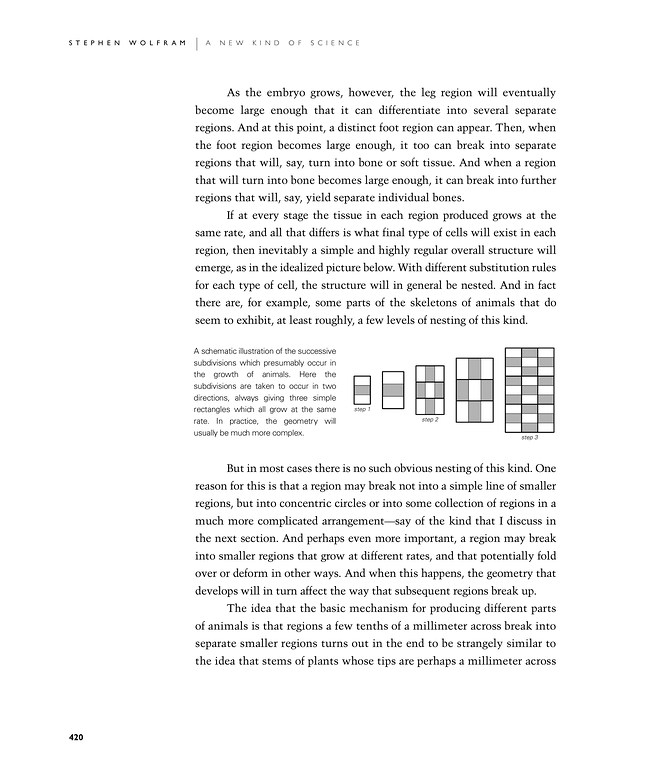
If at every stage the tissue in each region produced grows at the same rate, and all that differs is what final type of cells will exist in each region, then inevitably a simple and highly regular overall structure will emerge, as in the idealized picture below. With different substitution rules for each type of cell, the structure will in general be nested. And in fact there are, for example, some parts of the skeletons of animals that do seem to exhibit, at least roughly, a few levels of nesting of this kind.

But in most cases there is no such obvious nesting of this kind. One reason for this is that a region may break not into a simple line of smaller regions, but into concentric circles or into some collection of regions in a much more complicated arrangement—say of the kind that I discuss in the next section. And perhaps even more important, a region may break into smaller regions that grow at different rates, and that potentially fold over or deform in other ways. And when this happens, the geometry that develops will in turn affect the way that subsequent regions break up.

The idea that the basic mechanism for producing different parts of animals is that regions a few tenths of a millimeter across break into separate smaller regions turns out in the end to be strangely similar to the idea that stems of plants whose tips are perhaps a millimeter across

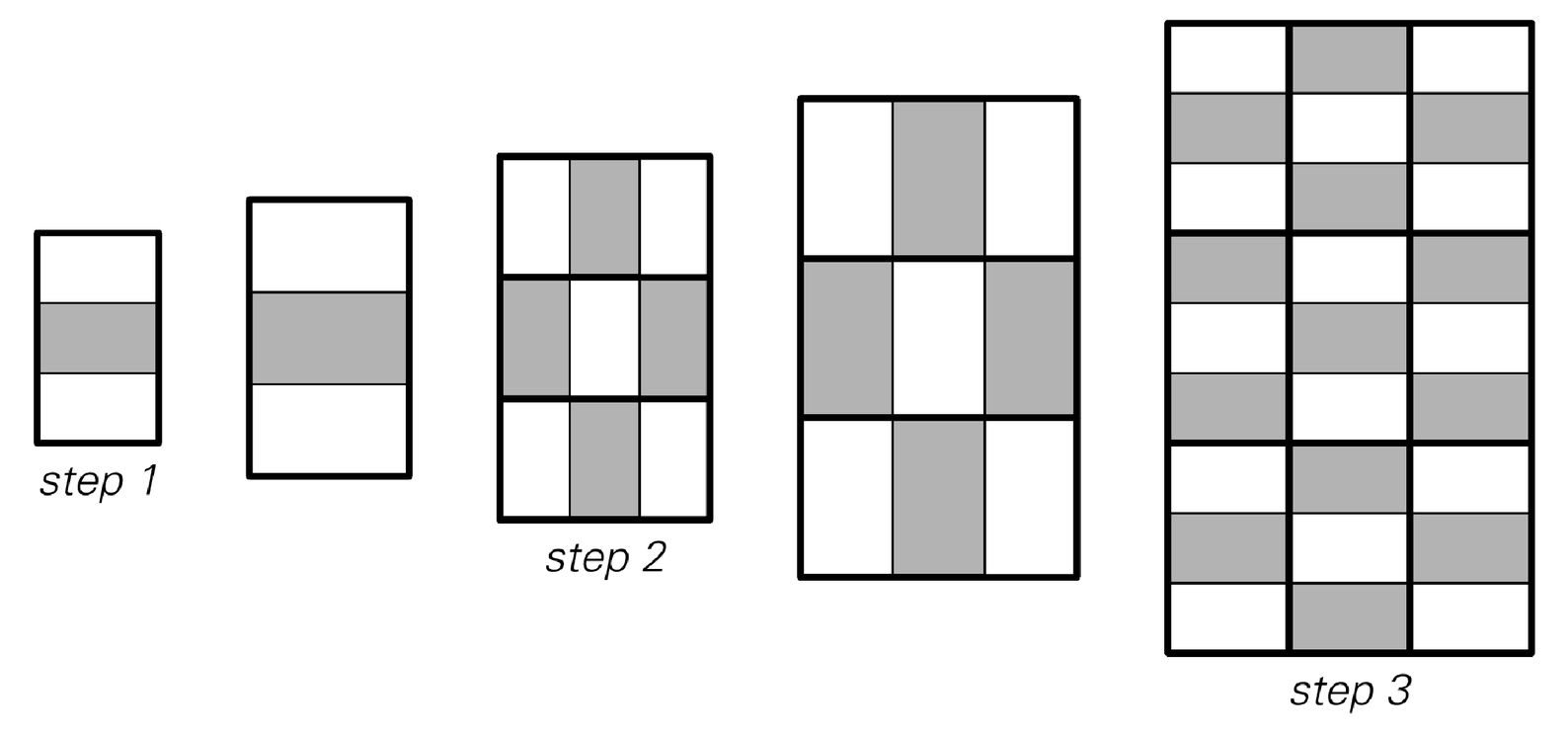
A schematic illustration of the successive subdivisions which presumably occur in the growth of animals. Here the subdivisions are taken to occur in two directions, always giving three simple rectangles which all grow at the same rate. In practice, the geometry will usually be much more complex.