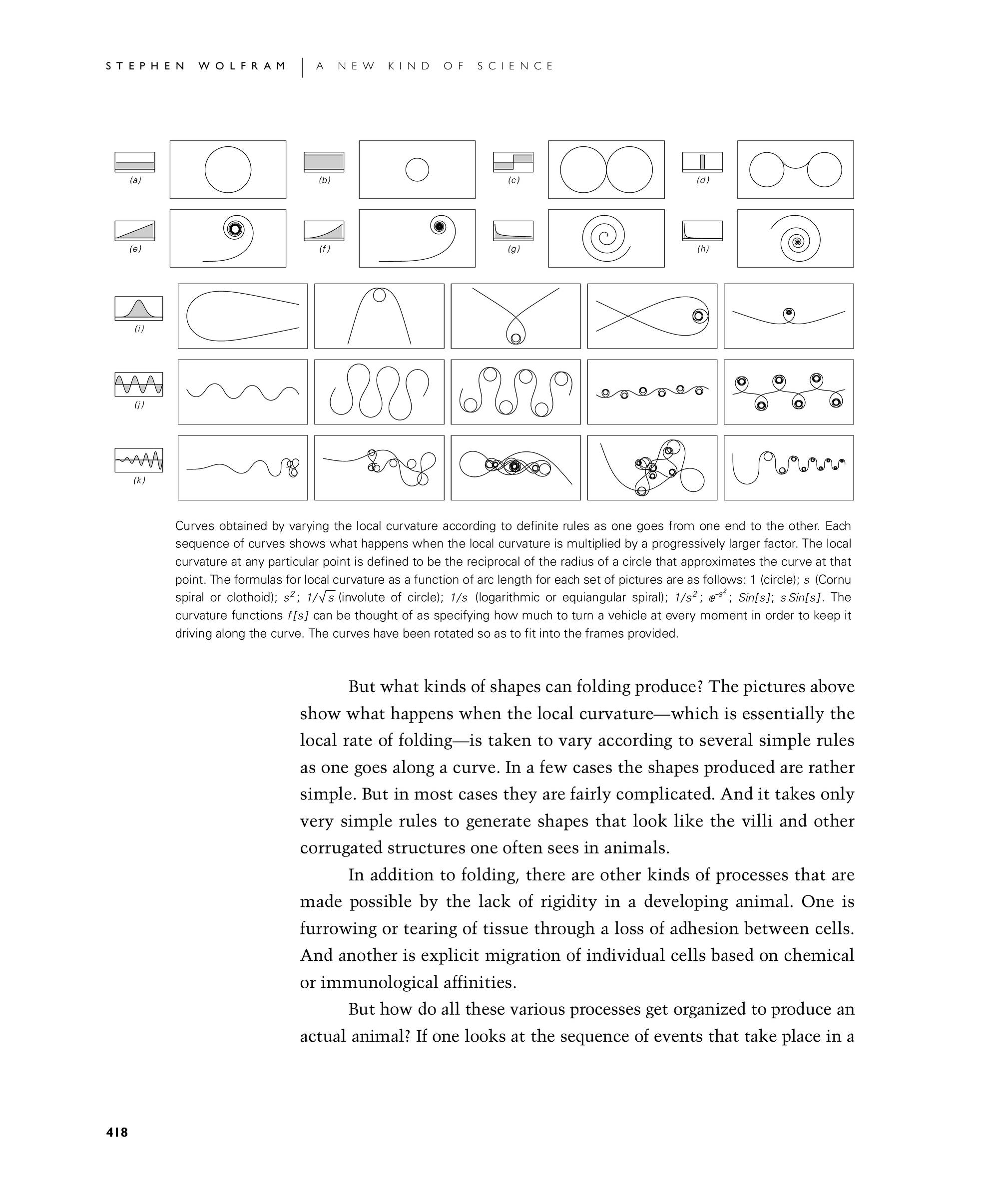
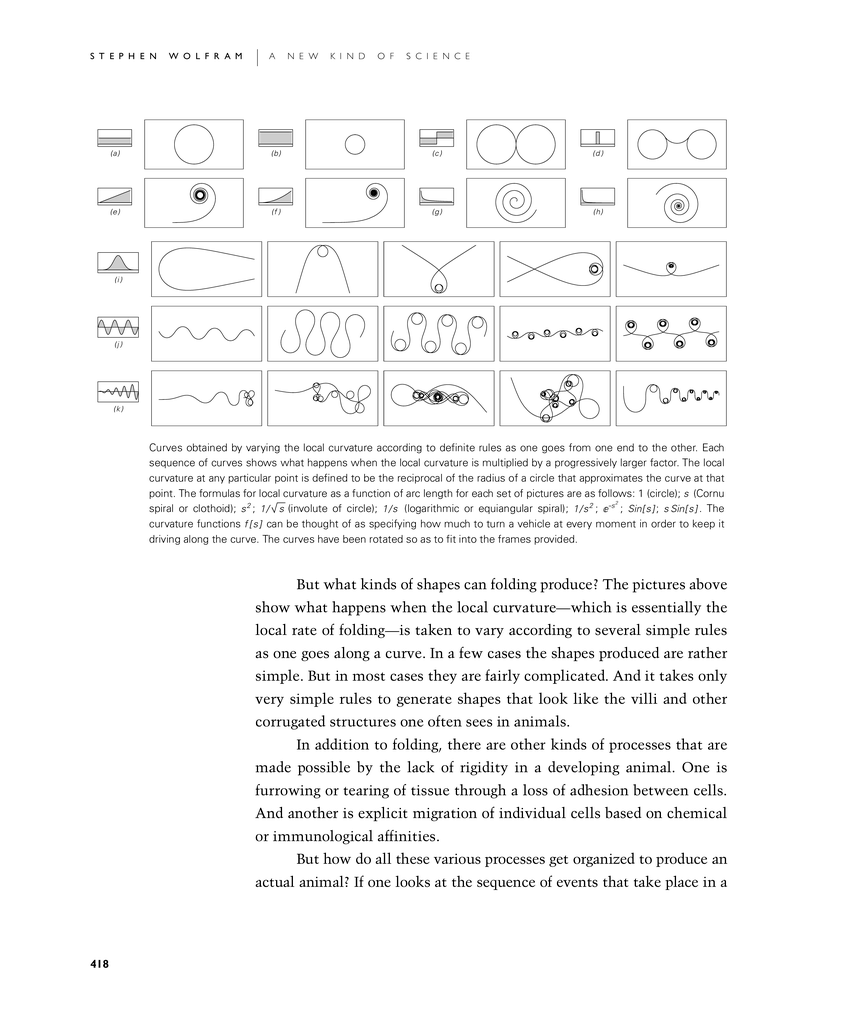
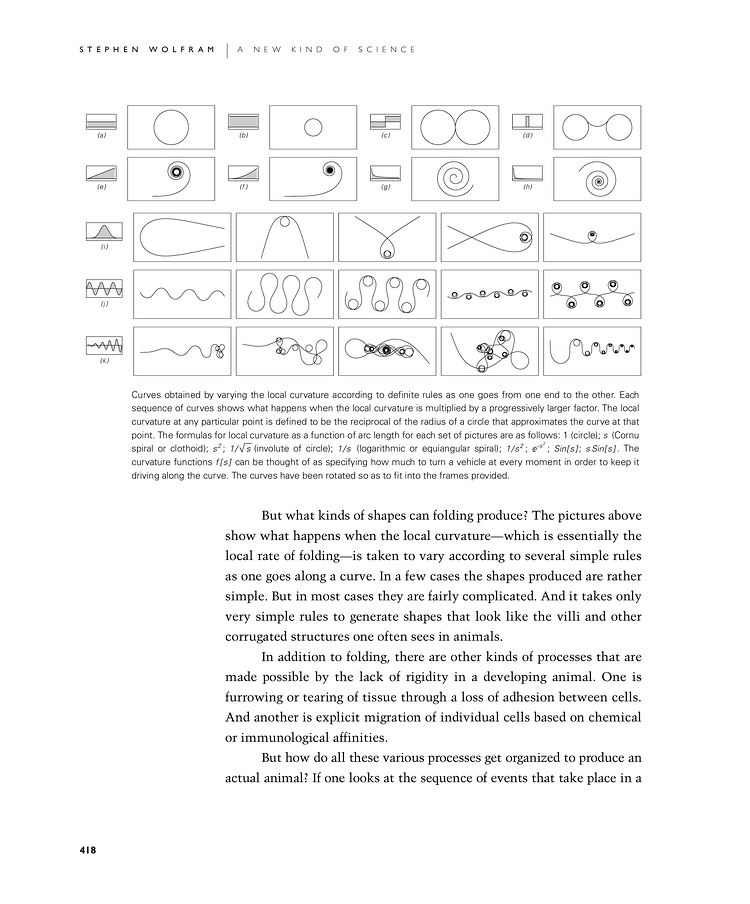
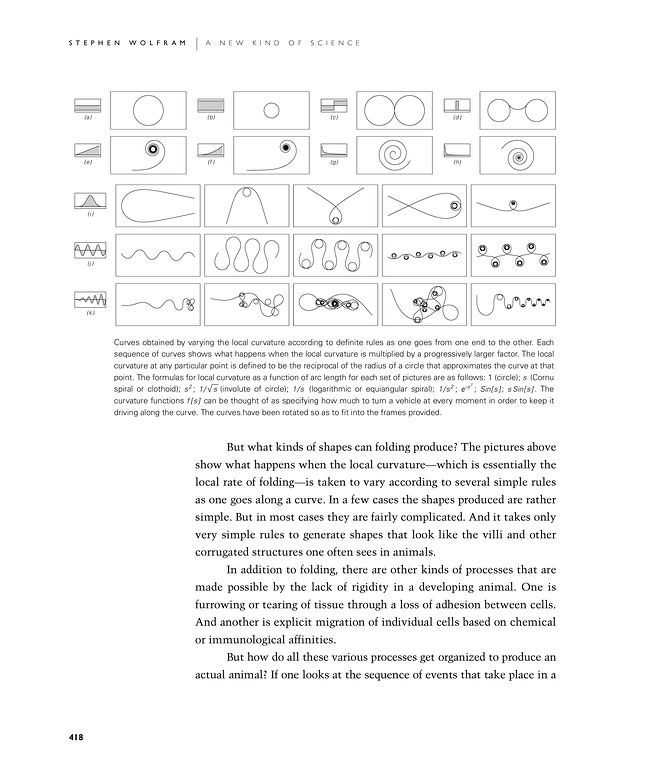
But what kinds of shapes can folding produce? The pictures below show what happens when the local curvature—which is essentially the local rate of folding—is taken to vary according to several simple rules as one goes along a curve. In a few cases the shapes produced are rather simple. But in most cases they are fairly complicated. And it takes only very simple rules to generate shapes that look like the villi and other corrugated structures one often sees in animals.
In addition to folding, there are other kinds of processes that are made possible by the lack of rigidity in a developing animal. One is furrowing or tearing of tissue through a loss of adhesion between cells. And another is explicit migration of individual cells based on chemical or immunological affinities.
But how do all these various processes get organized to produce an actual animal? If one looks at the sequence of events that take place in a
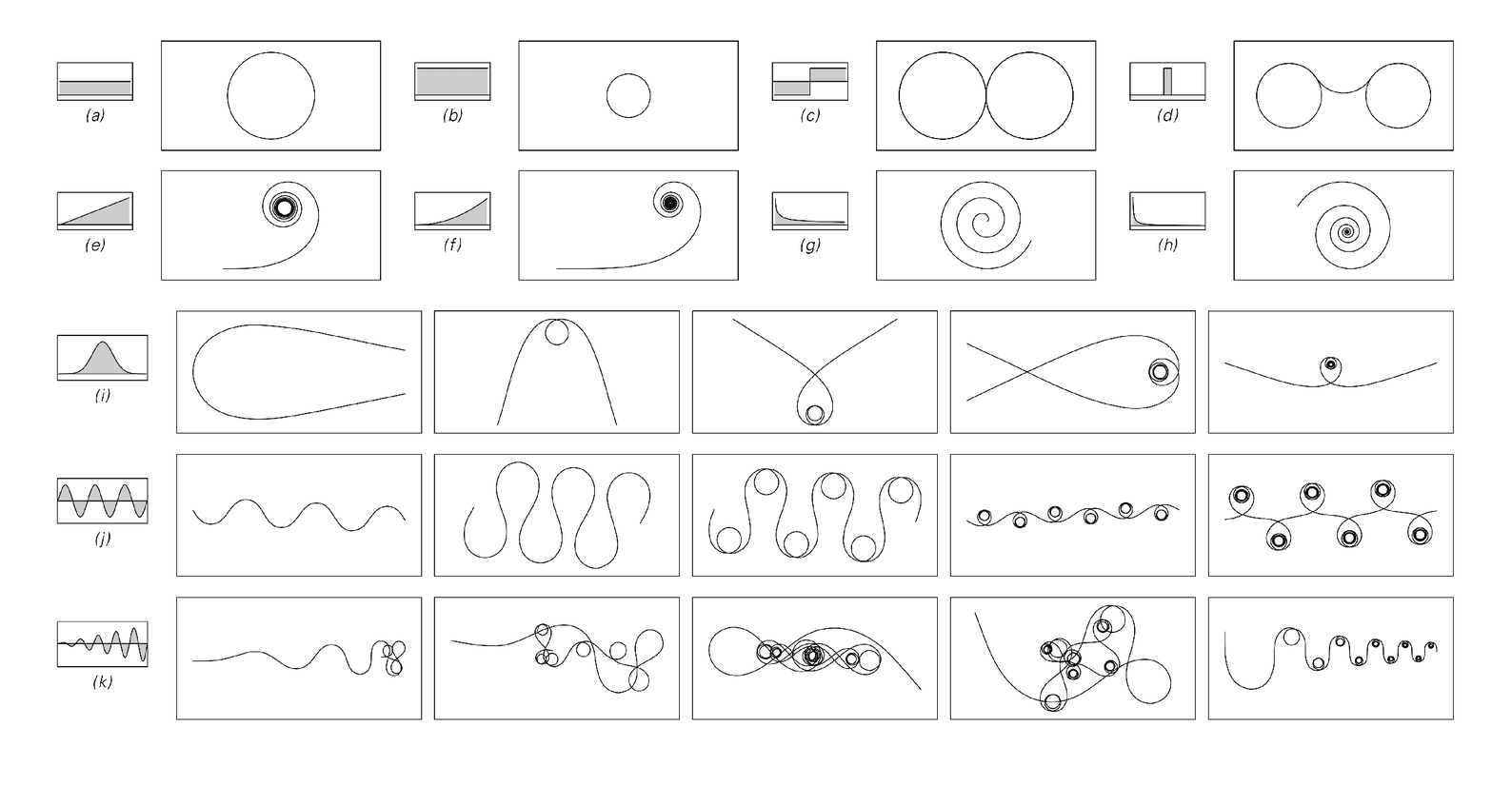
Curves obtained by varying the local curvature according to definite rules as one goes from one end to the other. Each sequence of curves shows what happens when the local curvature is multiplied by a progressively larger factor. The local curvature at any particular point is defined to be the reciprocal of the radius of a circle that approximates the curve at that point. The formulas for local curvature as a function of arc length for each set of pictures are as follows: 1 (circle); s (Cornu spiral or clothoid); s2; 1/Sqrt[s] (involute of circle); 1/s (logarithmic or equiangular spiral); 1/s2; Exp[-s2]; Sin[s]; s Sin[s]. The curvature functions f[s] can be thought of as specifying how much to turn a vehicle at every moment in order to keep it driving along the curve. The curves have been rotated so as to fit into the frames provided.