It is common for uniform behavior to be quite independent of initial conditions or other input to a system. But sometimes different uniform behavior can be obtained with different input.

One way this can happen, illustrated in the first set of pictures below, is for the system to conserve some quantity—such as total density of black—and for this quantity to end up being spread uniformly throughout the system by its evolution.

An alternative is that the system may always evolve to certain specific uniform phases, but the choice of which phase may depend on the total value of some quantity, as in the second set of pictures below.

Constraints are yet another basis for uniformity. And as a trivial example, the constraint in a line of black or white cells that every cell should be the same color as both its neighbors immediately implies that the whole line must be either uniformly black or uniformly white.

Beyond uniformity, repetition can be considered the next-simplest form of behavior. Repetition in time corresponds just to a system repeatedly returning to a particular state.

This can happen if, for example, the behavior of a system in effect follows some closed curve such as a circle which always leads back to the same point. And in general, in any system with definite rules that only ever visits a limited number of states, it is

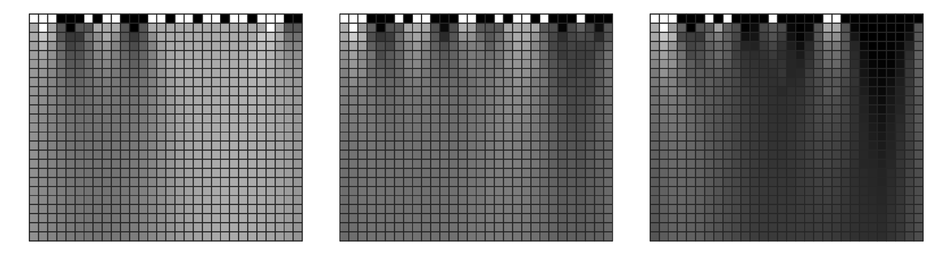
With each cell at each step having a gray level that is the average of its predecessor and its two neighbors the total amount of black is conserved, but eventually becomes spread uniformly throughout the system.

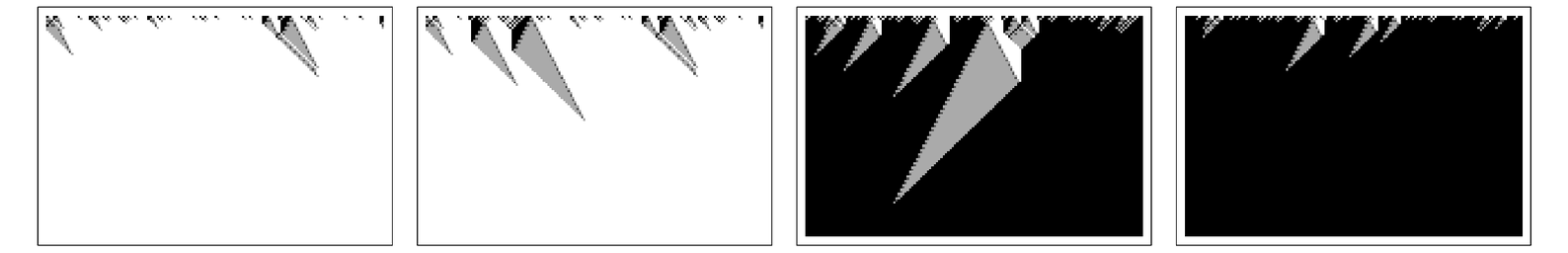
With different initial conditions this cellular automaton from page 339 can evolve either to uniform white or uniform black. Such discrete transitions are somewhat less common in one dimension than elsewhere.