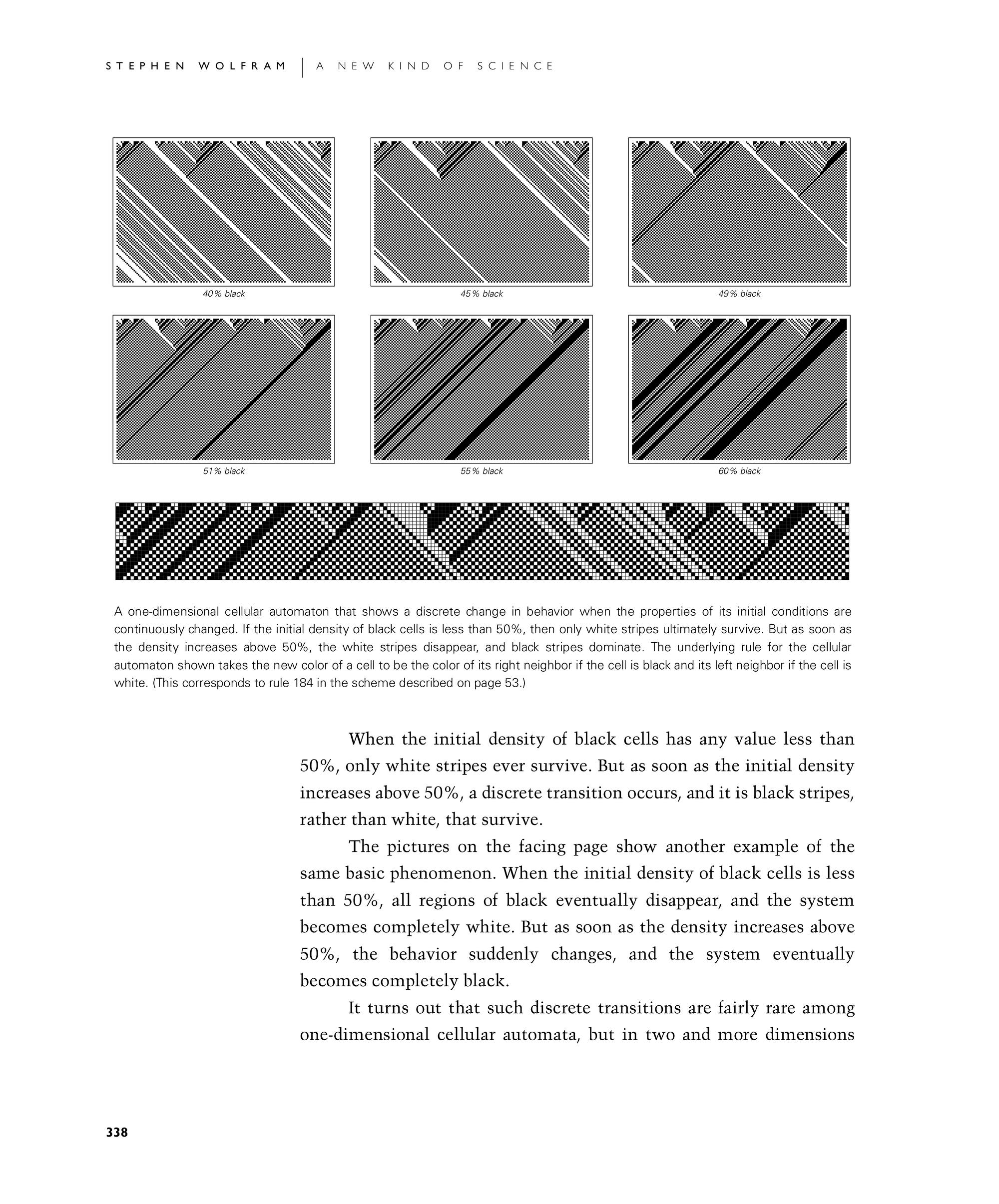
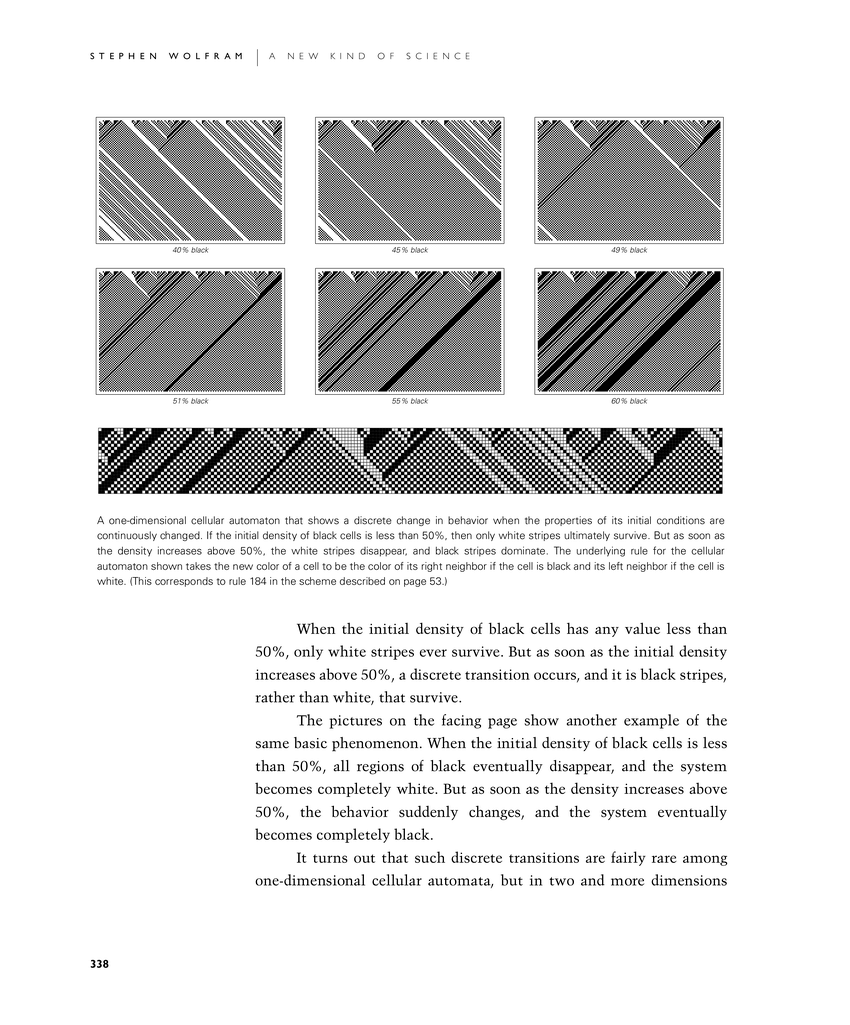
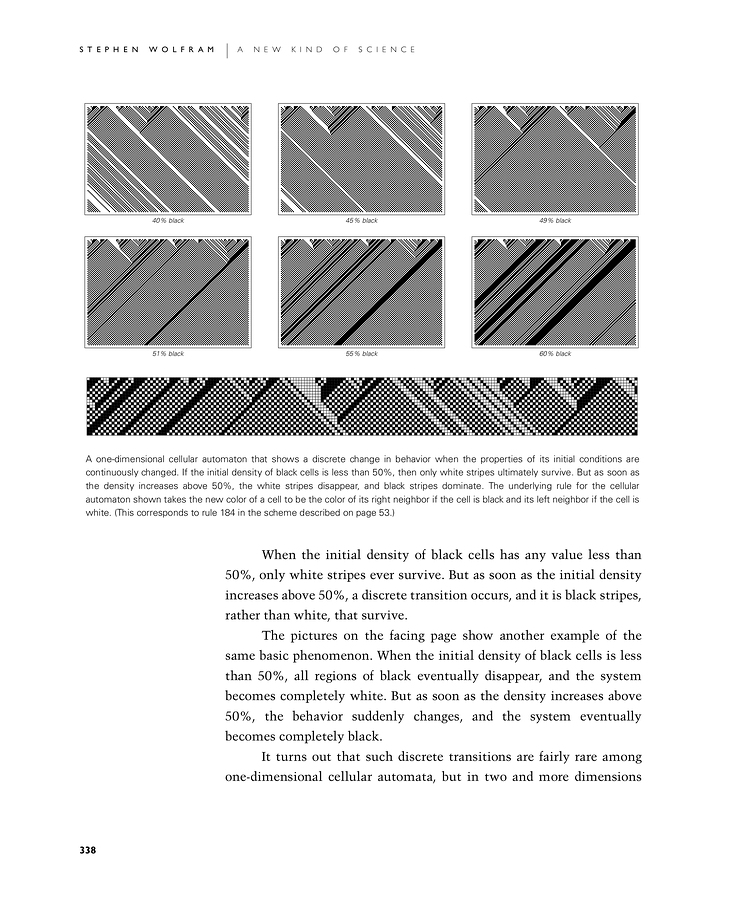
When the initial density of black cells has any value less than 50%, only white stripes ever survive. But as soon as the initial density increases above 50%, a discrete transition occurs, and it is black stripes, rather than white, that survive.

The pictures on the facing page show another example of the same basic phenomenon. When the initial density of black cells is less than 50%, all regions of black eventually disappear, and the system becomes completely white. But as soon as the density increases above 50%, the behavior suddenly changes, and the system eventually becomes completely black.

It turns out that such discrete transitions are fairly rare among one-dimensional cellular automata, but in two and more dimensions

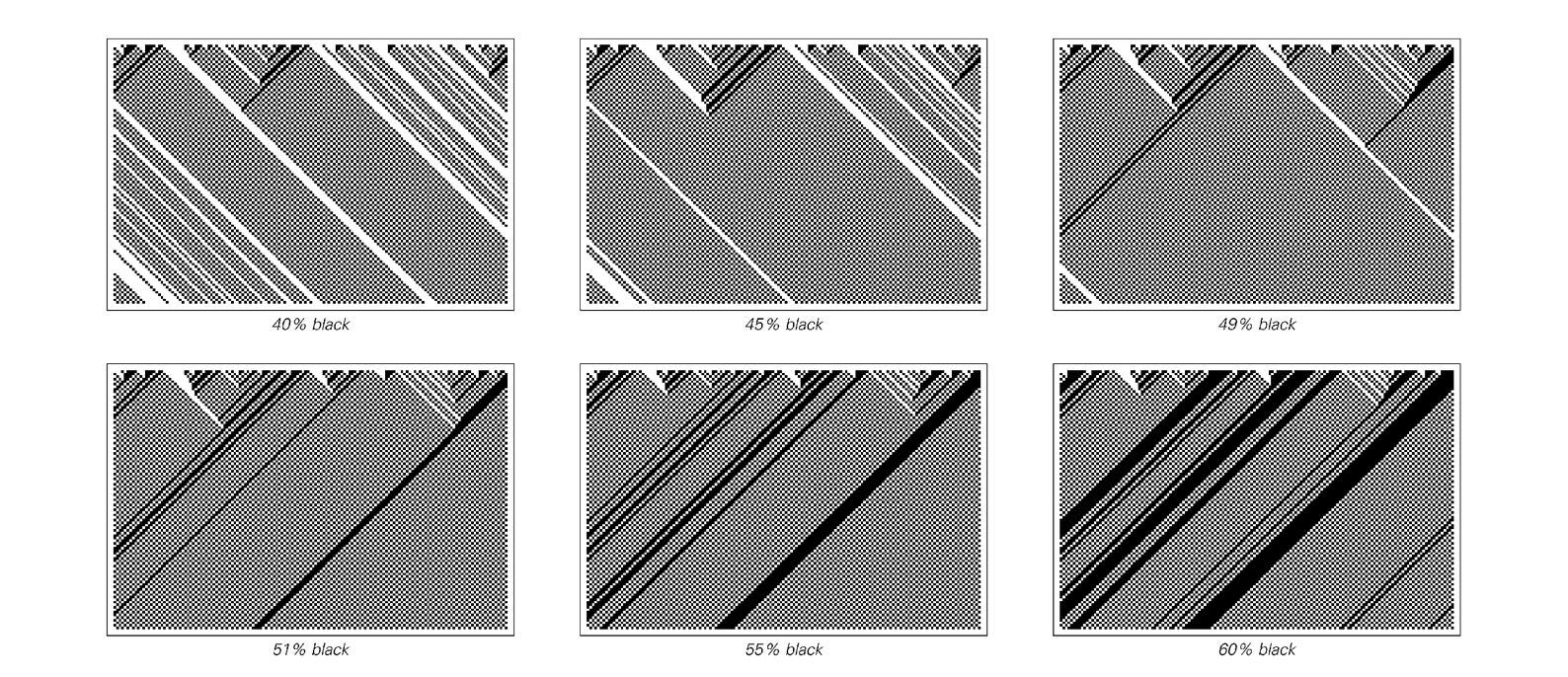
A one-dimensional cellular automaton that shows a discrete change in behavior when the properties of its initial conditions are continuously changed. If the initial density of black cells is less than 50%, then only white stripes ultimately survive. But as soon as the density increases above 50%, the white stripes disappear, and black stripes dominate. The underlying rule for the cellular automaton shown takes the new color of a cell to be the color of its right neighbor if the cell is black and its left neighbor if the cell is white. (This corresponds to rule 184 in the scheme described on page 53.)