further. And then in the first case, the subsequent motion looks quite random. But in the second case it is fairly regular. So why is this?

A little analysis shows what is going on. The basic idea is to represent the position of each point at each step as a number, say x, which runs from 0 to 1. When the material is stretched, the number is

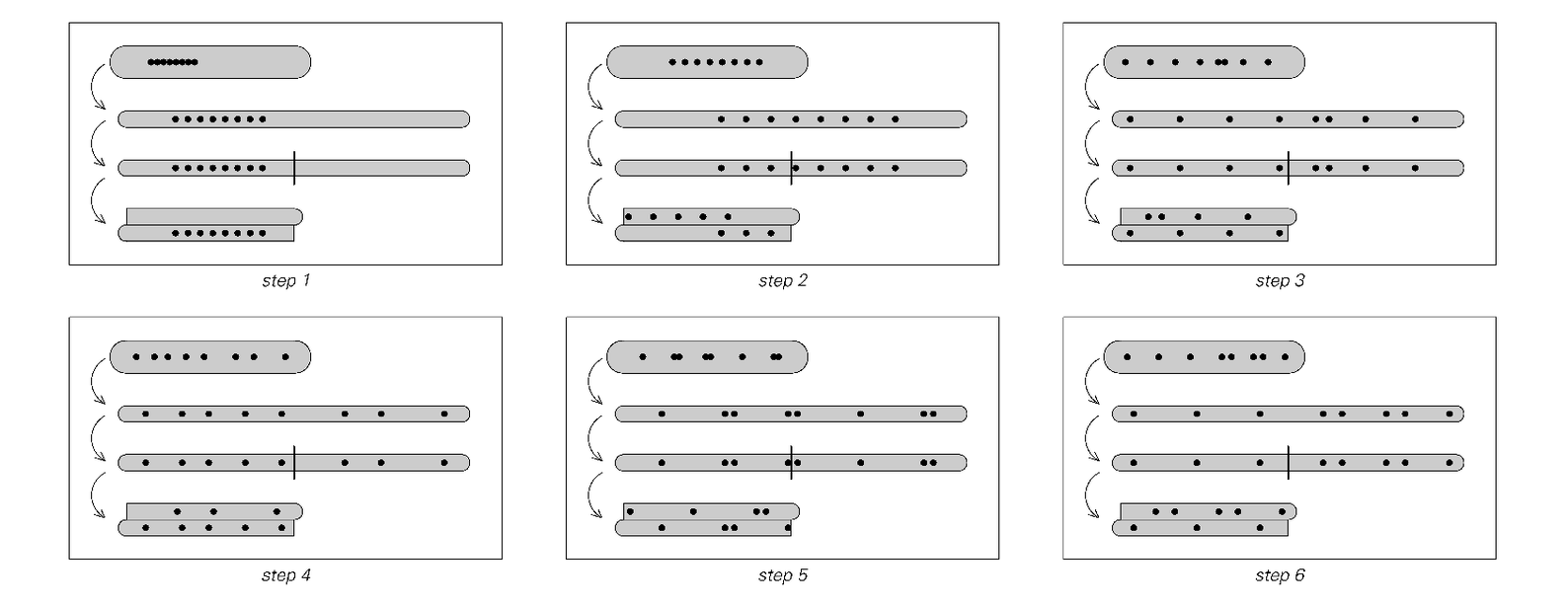
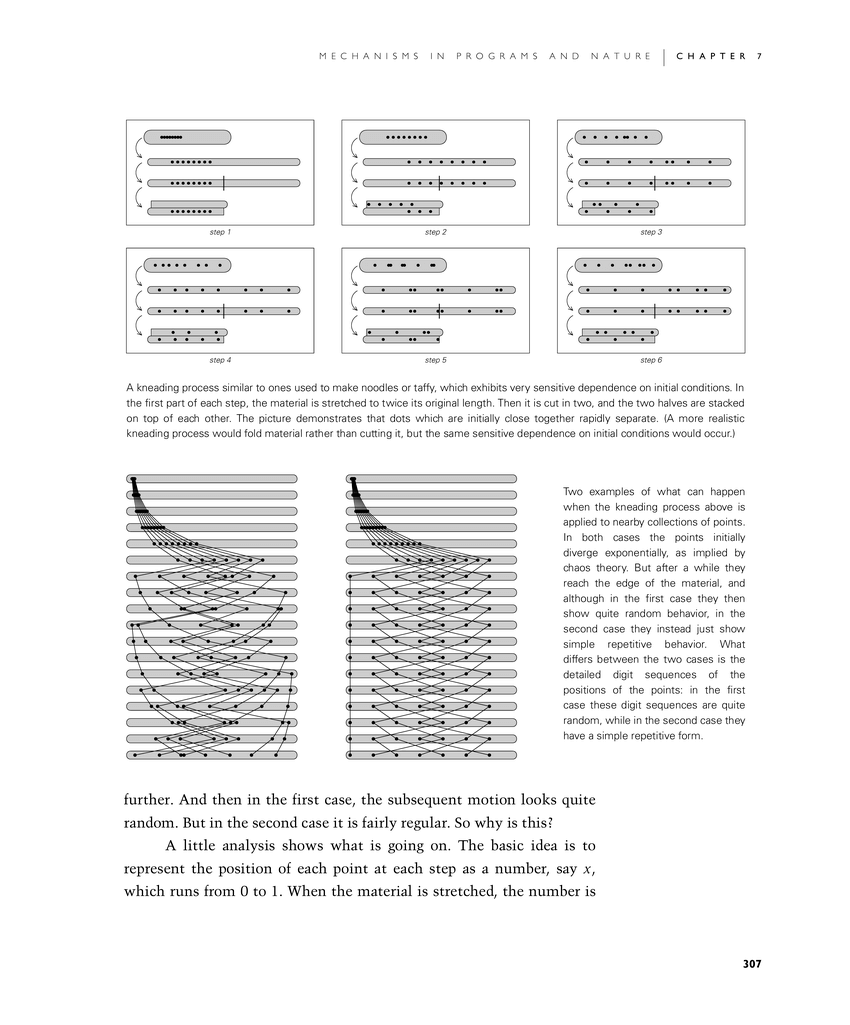
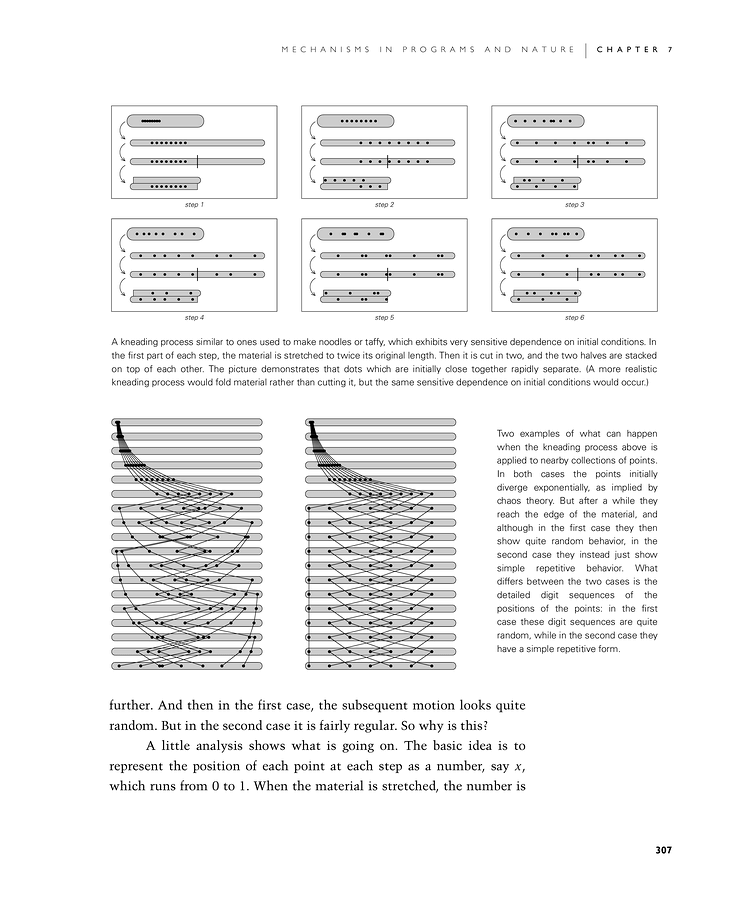
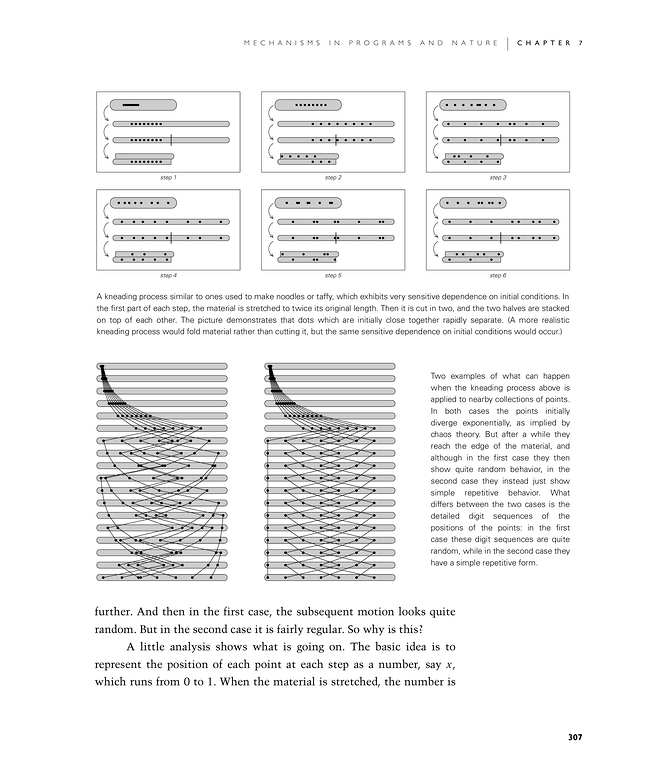
A kneading process similar to ones used to make noodles or taffy, which exhibits very sensitive dependence on initial conditions. In the first part of each step, the material is stretched to twice its original length. Then it is cut in two, and the two halves are stacked on top of each other. The picture demonstrates that dots which are initially close together rapidly separate. (A more realistic kneading process would fold material rather than cutting it, but the same sensitive dependence on initial conditions would occur.)

Two examples of what can happen when the kneading process above is applied to nearby collections of points. In both cases the points initially diverge exponentially, as implied by chaos theory. But after a while they reach the edge of the material, and although in the first case they then show quite random behavior, in the second case they instead just show simple repetitive behavior. What differs between the two cases is the detailed digit sequences of the positions of the points: in the first case these digit sequences are quite random, while in the second case they have a simple repetitive form.