Randomness in Class 3 Systems

When one looks at class 3 systems the most obvious feature of their behavior is its apparent randomness. But where does this randomness ultimately come from? And is it perhaps all somehow just a reflection of randomness that was inserted in the initial conditions?

The presence of randomness in initial conditions—together with sensitive dependence on initial conditions—does imply at least some degree of randomness in the behavior of any class 3 system. And indeed when I first saw class 3 cellular automata I assumed that this was the basic origin of their randomness.

But the crucial point that I discovered only some time later is that random behavior can also occur even when there is no randomness in initial conditions. And indeed, in earlier chapters of this book we have already seen many examples of this fundamental phenomenon.

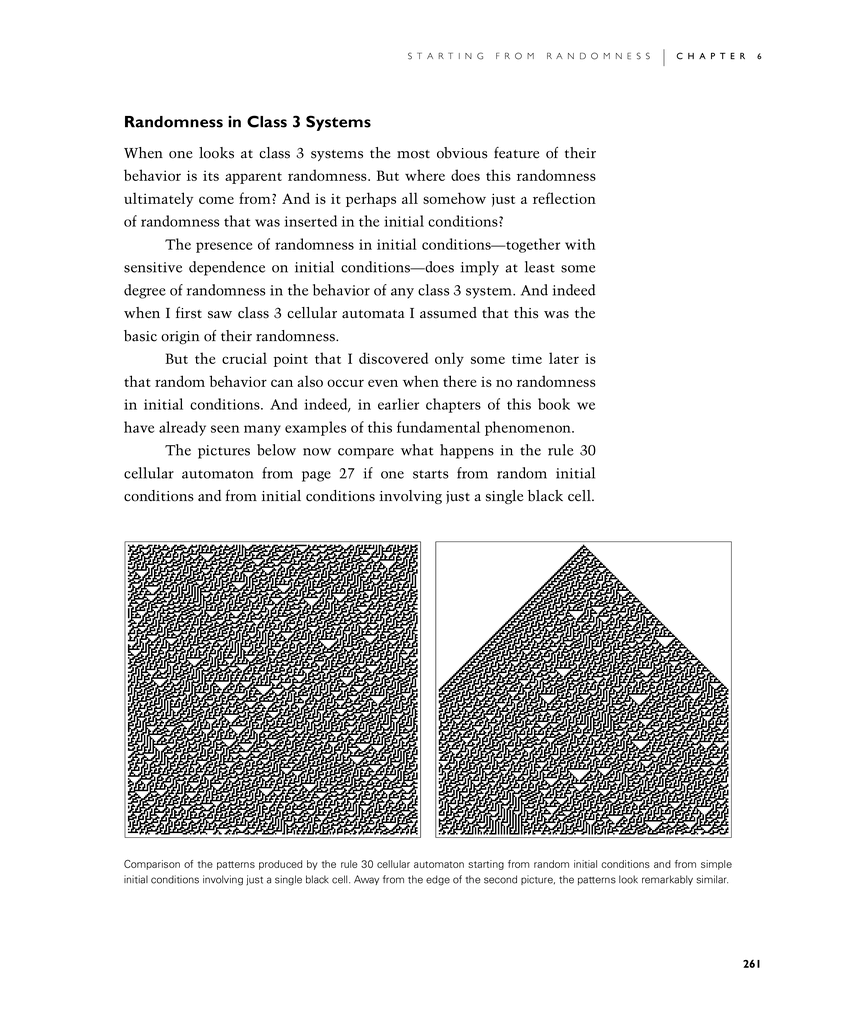
The pictures below now compare what happens in the rule 30 cellular automaton from page 27 if one starts from random initial conditions and from initial conditions involving just a single black cell.

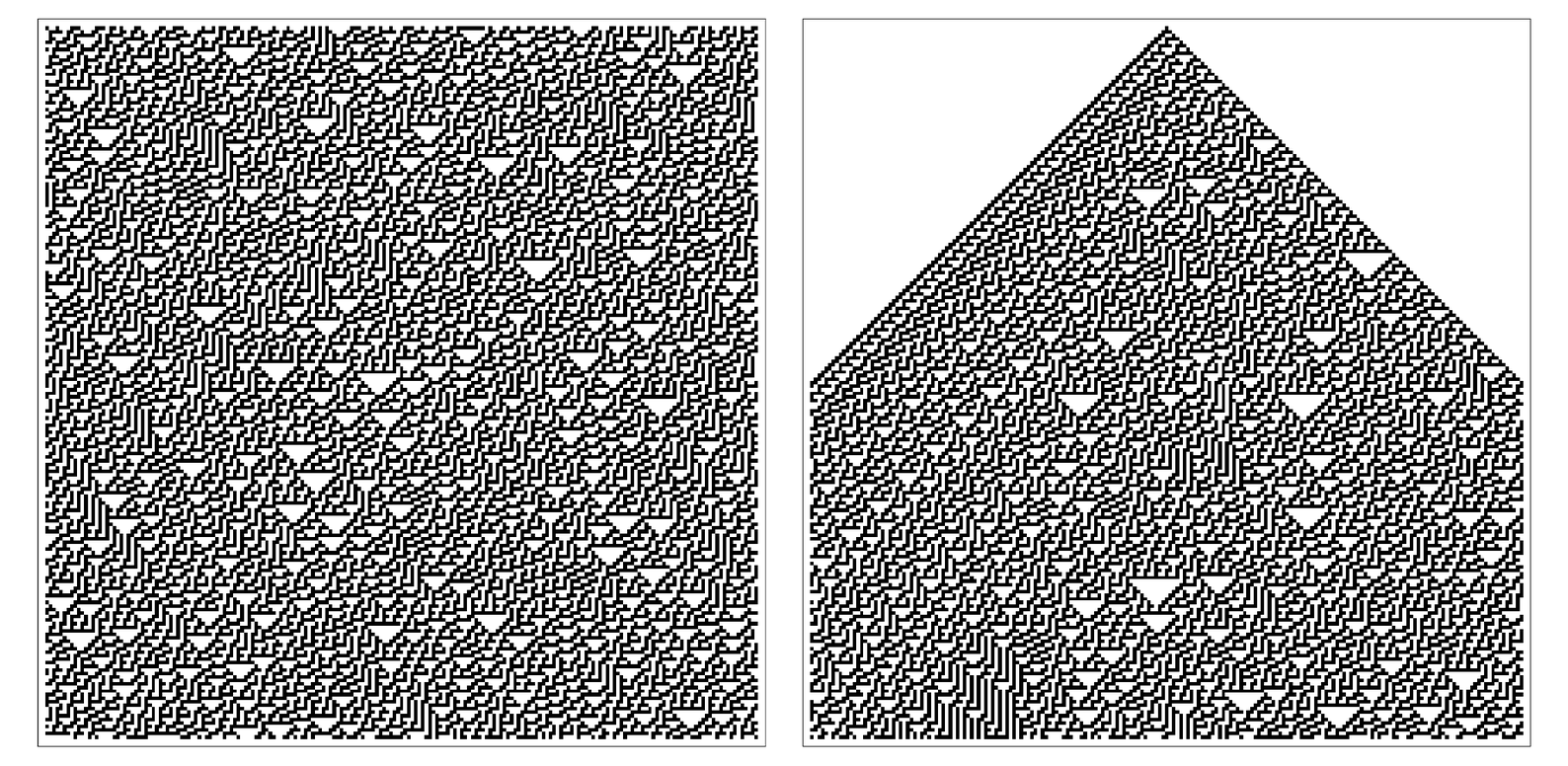
Comparison of the patterns produced by the rule 30 cellular automaton starting from random initial conditions and from simple initial conditions involving just a single black cell. Away from the edge of the second picture, the patterns look remarkably similar.