same way, so that it ultimately evolves to produce an identical copy of the whole pattern.

But in fact there is nothing about this basic process that depends on the squares being arranged in any kind of rigid grid. And the picture below shows what happens if one just uses a simple geometrical rule to replace each black square by two smaller black squares. The result, once again, is that one gets an intricate but highly regular nested pattern.

In a substitution system where black squares are arranged on a grid, one can be sure that different squares will never overlap. But if there is just a geometrical rule that is used to replace each black square, then it is possible for the squares produced to overlap, as in the picture on the next page. Yet at least in this example, the overall pattern that is ultimately obtained still has a purely nested structure.

The general idea of building up patterns by repeatedly applying geometrical rules is at the heart of so-called fractal geometry. And the

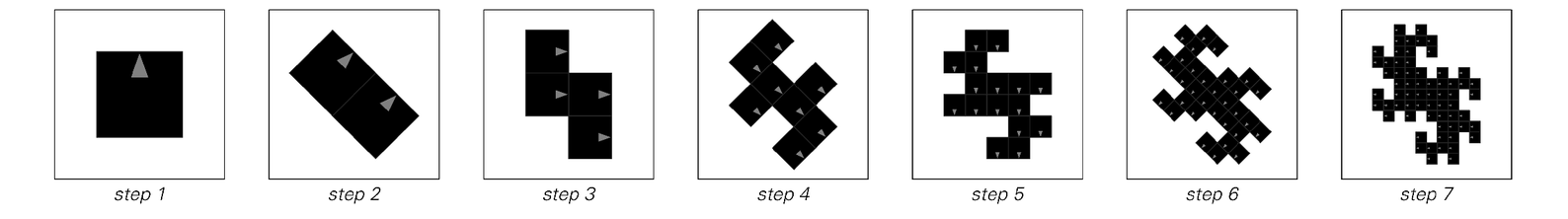
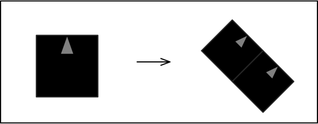
The pattern obtained by starting with a single black square and then at every step replacing each black cell with two smaller black cells according to the simple geometrical rule shown on the left. Note that in applying the rule to a particular square, one must take account of the orientation of that square. The final pattern obtained has an intricate nested structure.