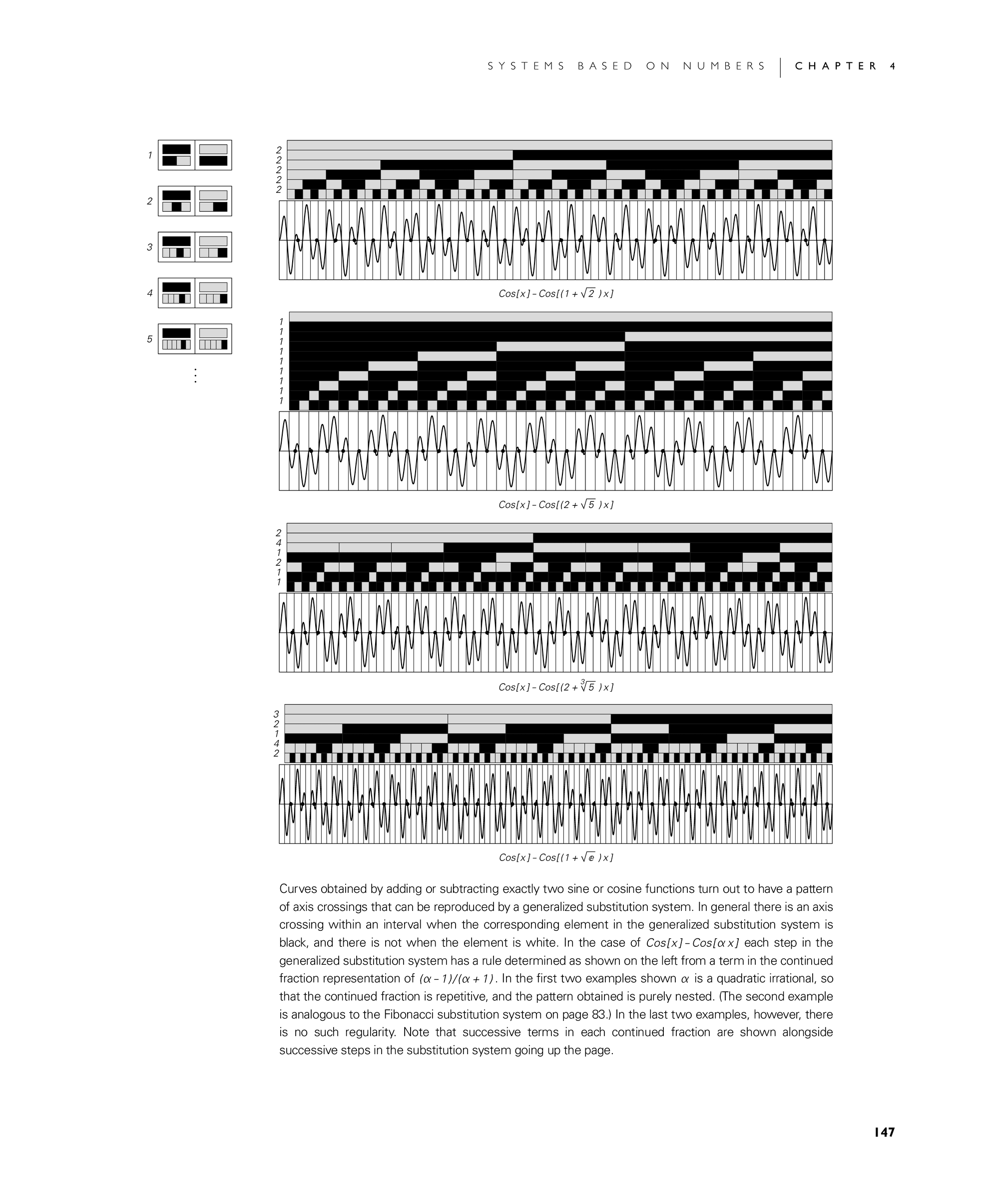
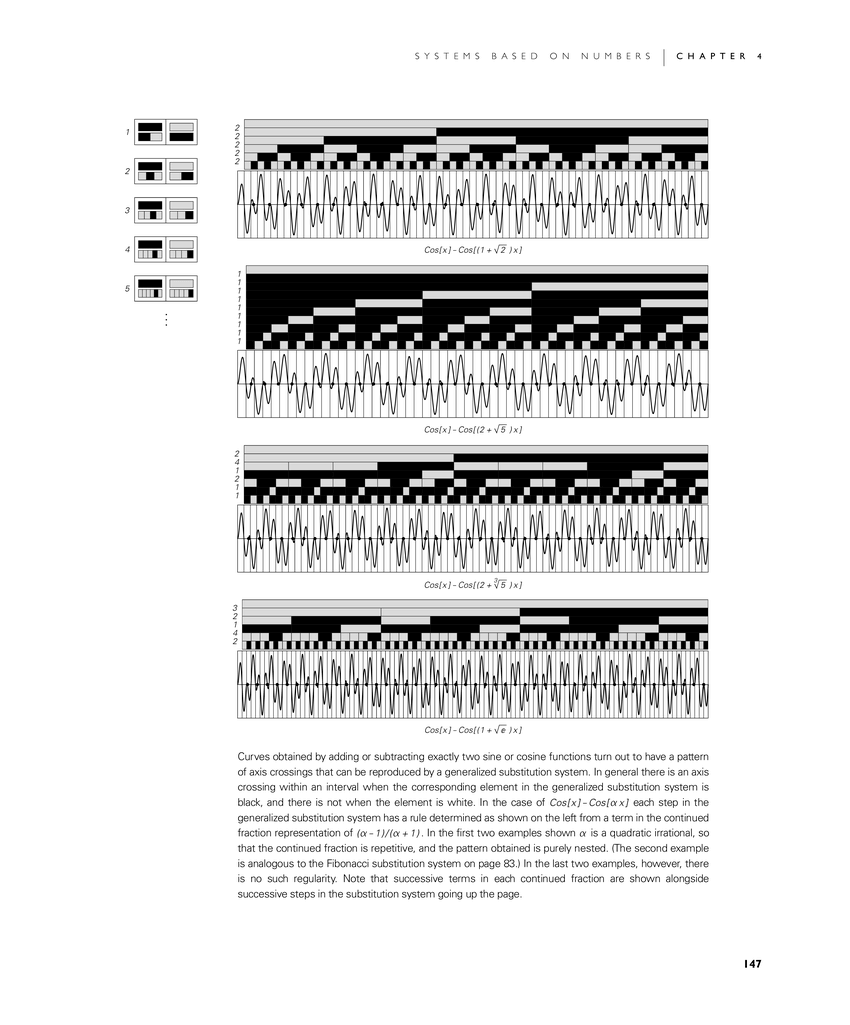
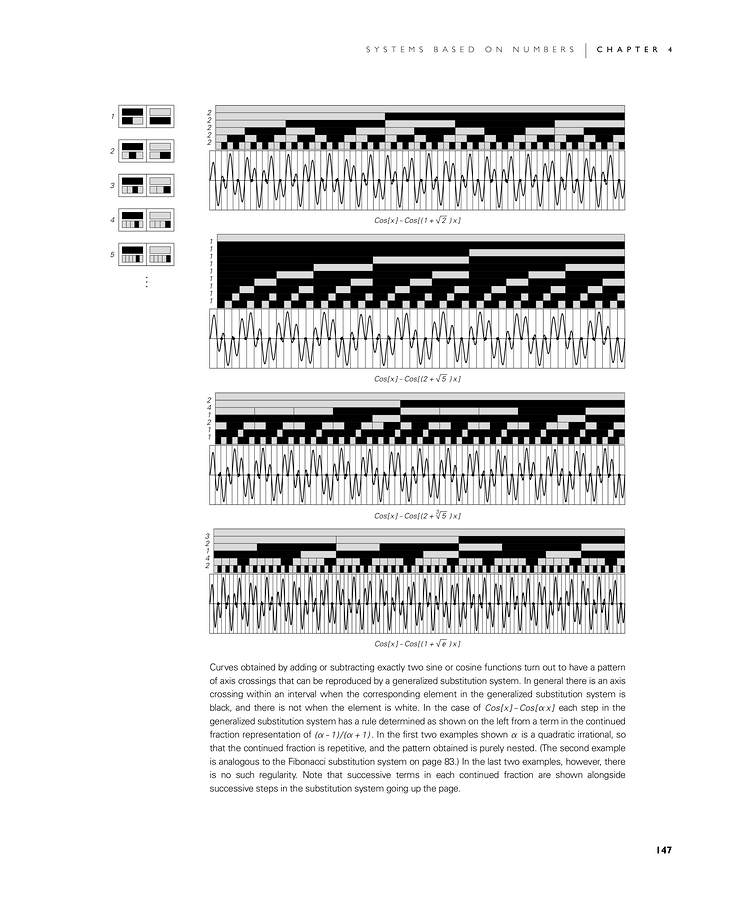
Curves obtained by adding or subtracting exactly two sine or cosine functions turn out to have a pattern of axis crossings that can be reproduced by a generalized substitution system. In general there is an axis crossing within an interval when the corresponding element in the generalized substitution system is black, and there is not when the element is white. In the case of Cos[x] – Cos[α x] each step in the generalized substitution system has a rule determined as shown on the left from a term in the continued fraction representation of (α–1)/(α+1). In the first two examples shown α is a quadratic irrational, so that the continued fraction is repetitive, and the pattern obtained is purely nested. (The second example is analogous to the Fibonacci substitution system on page 83.) In the last two examples, however, there is no such regularity. Note that successive terms in each continued fraction are shown alongside successive steps in the substitution system going up the page.